As the title says, I'm looking for the marginal densities of $$f (x,y) = c \sqrt{1 x^2 y^2}, x^2 y^2 \leq 1$$ So far I have found $c$ to be $\frac{3}{2 \pi}$ I figured that out through converting $f(x,y)$ into polar coordinates and integrating over $drd\theta$, which is why I'm stuck on the marginal densities portionS is defined as a sphere However, when I type "S f(x,y,z) = 1" into the input bar, nothing is graphed and the algebra window shows S as an undefined Implicit Curve I need to keep the function f, soThanks 13 comments share save hide report 100% Upvoted This thread is archived New comments cannot be posted and votes cannot be cast Sort by best level 1 5 years ago So for domain, we have that x 2
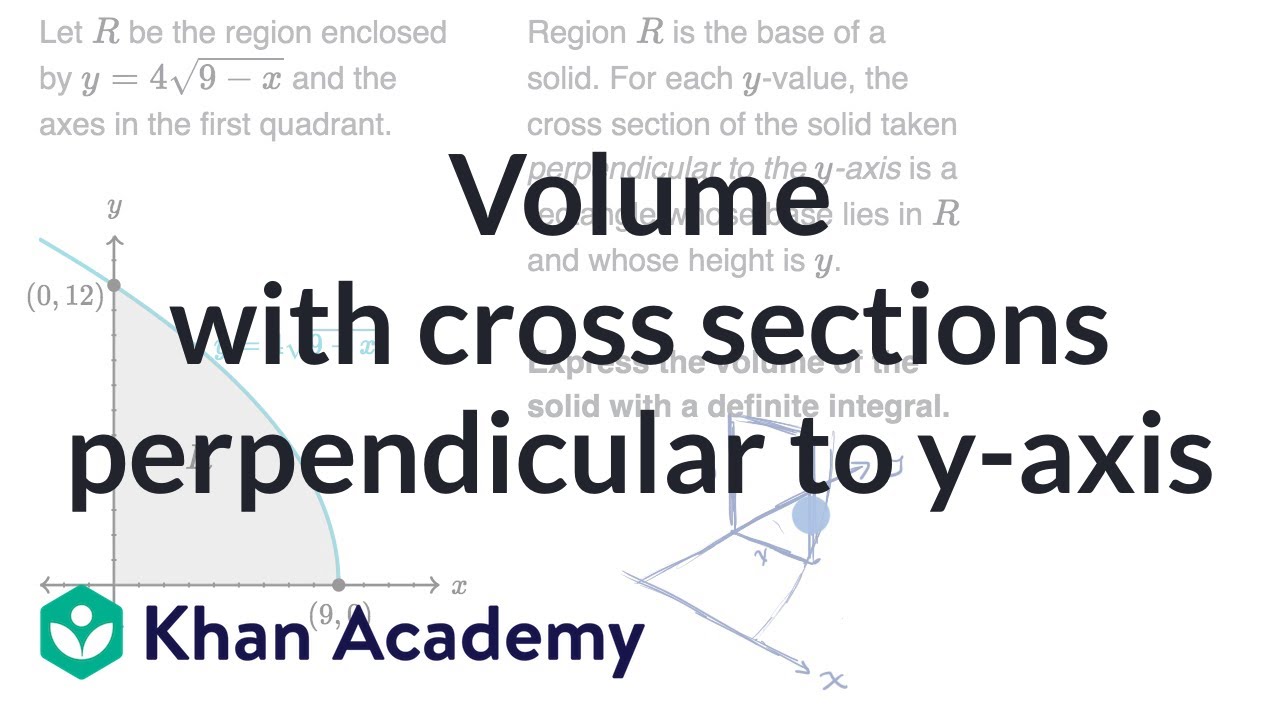
Volume With Cross Sections Perpendicular To Y Axis Video Khan Academy
F(x y)=sqrt(4x^2+y^2)
F(x y)=sqrt(4x^2+y^2)-Experts are waiting 24/7 to provide stepbystep solutions in as fast as 30 minutes!* See Answer *Response times vary by subjectDer Arkussinus – geschrieben oder – und der Arkuskosinus (oder auch Arkuscosinus) – geschrieben oder – sind Umkehrfunktionen der (geeignet) eingeschränkten Sinus bzw KosinusfunktionSinus und Kosinus sind Funktionen, die einen Winkel auf einen Wert im Intervall , abbilden;



Volume Of Region Bounded By Z 4 Sqrt X 2 Y 2 And Z Sqrt X 2 Y 2 Mathematics Stack Exchange
Log in here Relevant For Algebra > Common Misconceptions (Algebra) Nihar Mahajan, A Former Brilliant Member, Zandra Vinegar, and 2 others Eli Ross Jimin Khim contributed This is part of a series on common misconceptions Is this conception true orO gráfico de $f(x,y) = g(\sqrt{x^{2} y^{2}})$ pode ser obtido rotacionando o gráfico de $g$ no plano $xz$ ao redor do eixo $z$Since y^2 = x − 2 is a relation (has more than 1 yvalue for each xvalue) and not a function (which has a maximum of 1 yvalue for each xvalue), we need to split it into 2 separate functions and graph them together So the first one will be y 1 = √(x − 2) and the second one is y 2 = −√(x − 2) When you graph these on the same axis
Here is another way to prove the continuity of f ( x, y) at ( 0, 0) x y x 2 y 2 − 0 = x y x 2 y 2 < x 2 y 2 x 2 y 2 x 2 y 2 = x 2 y 2 < ε ( where ε is a preassigned positive number) if x 2 y 2 < δ 2, where δ = ε So,given any ε > 0, ∃ δ > 0 such thatX 2 y 2 = x y?F (x,y)=cases (xy/ (x^2y^2), (x,y)!= (0,0);0, (x,y)= (0,0)) Meine Lösung Stetigkeit im Punkt (0,0) heißt \forall\ \epsilon>0 \exists\ \delta = \delta (\epsilon)>0 \abs ( (x,y), (0,0)) \abs (f (x,y)f (0,0))
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music WolframAlpha brings expertlevel knowledge andAlso does anyone know what this particular type of problem is called so I can research it?Does x 2 y 2 = x y?



The Area Of The Domain Of The Function F X Y Sqrt 16 X 2 Y 2



Implicit And Logarithmic Differentiation
2605 · Example 3 Identify the level curves of \(f\left( {x,y} \right) = \sqrt {{x^2} {y^2}} \) Sketch a few of them Show Solution First, for the sake of practice, let's identify what this surface given by \(f\left( {x,y} \right)\) is To do this let's rewrite it as, \z = \sqrt {{x^2} {y^2}} \ Recall from the Quadric Surfaces section that this the upper portion of the "cone" (or hourSolution for Suppose f(x,y,z)=1/(sqrt(x^2y^2z^2)) and W is the bottom half of a sphere of radius 6 Enter ρ as rho, ϕ as phi, and θ as theta What are theVectors play an important role in physics, engineering, and mathematics In mathematics, a vector (from the Latin "mover") is a geometric object that has a magnitude (or length) and a direction Vectors can be added to other vectors according to vector algebra, and can be multiplied by a scalar (real number)
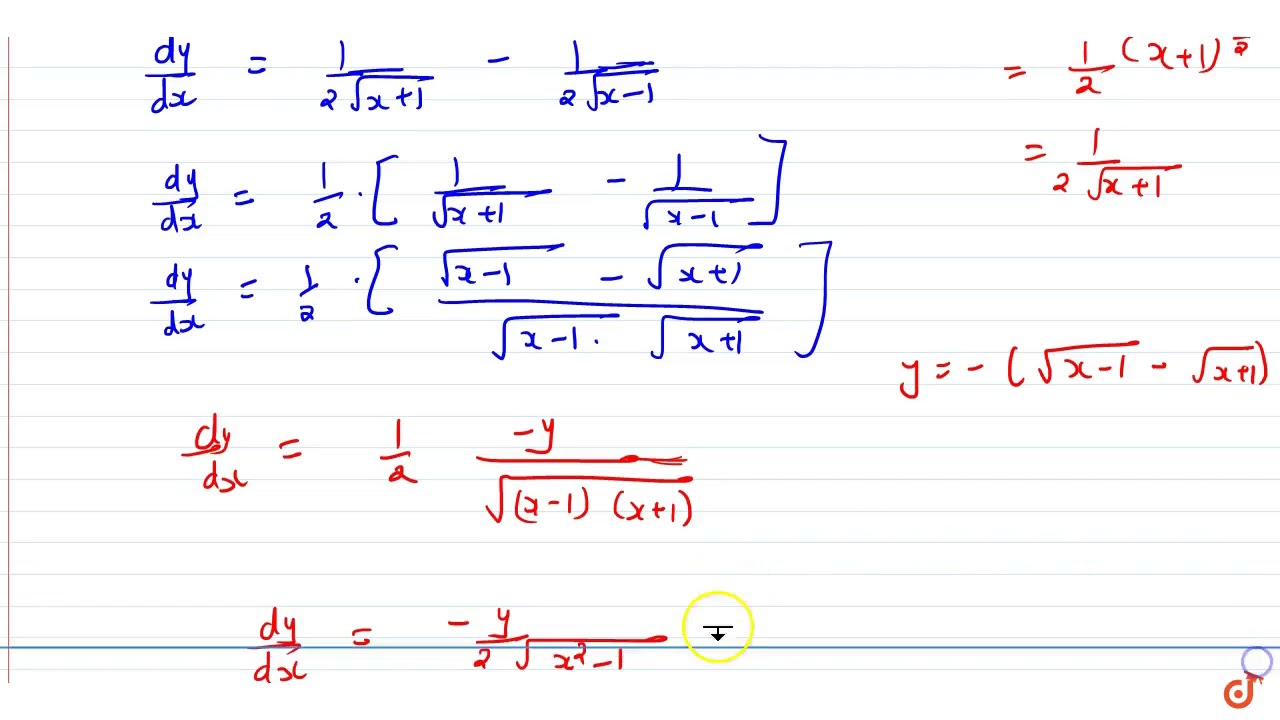


If Y Sqrt X 1 Sqrt X 1 Prove That X 2 1 D 2y Dx 2 X Dy Dx Y 4 0 Youtube
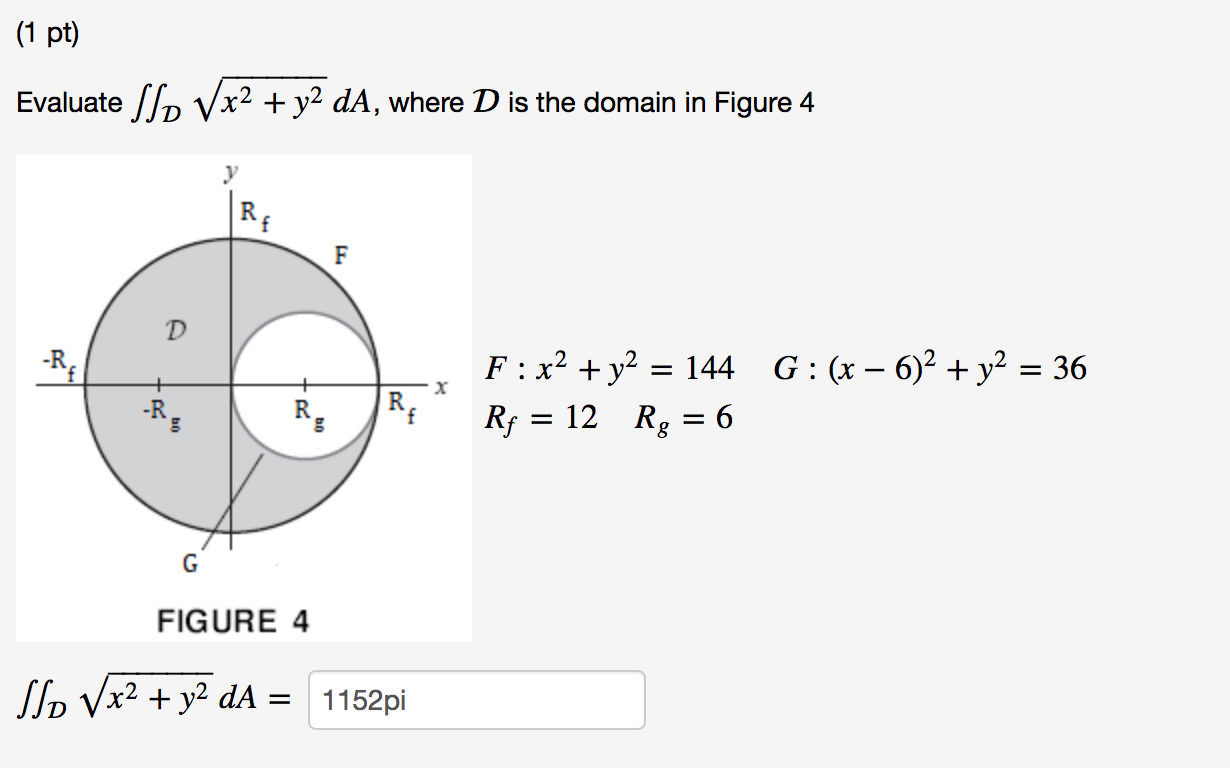


Solved Evaluate Double Integral D Square Root X 2 Y 2 Chegg Com
See Answer Check out a sample Q&A here Want to see this answer and more?Specify Method (new) Chain Rule;Ich habe eine Frage zu folgender Aufgabe Untersuchen sie die Stetigkeit von f\IR^2>\IR im Punkt (0,0)!



Xy Partial Derivative Page 1 Line 17qq Com


Solved 1 What Can Be Said About The Domain Of The Functionf0gwheref Y 4 Y 2and G X 5 3x 1 Course Hero
I have a function f(x,y,z) = x^2 y^2 z^2 and I'd like to graph the surface defined by the equation f(x,y,z) = 1 When I type "S x^2 y^2 z^2 = 1" into the input bar, this works perfectly;Find and sketch the domain of f(x, y) = (sqrt(x^2 y^2 1) ln(4 x^2 y^2))/(sqrt(x y))F = @(x,y) sqrt(x^2 y^2);



14 2 Limits And Continuity



Solved Find The Gradient Vector Field Of F X Y Square Chegg Com
Apr 14, 05 #1 VinnyCee 4 0 Here is the problem First Part (already done) Find the volume of the solid that is bounded above by the cylinder texz = 4 x^2/tex, on the sides by the cylinder texx^2 y^2 = 4/tex, and below byQuestion Graph F(x Y)=sqrt(9x^2y^2) This problem has been solved!Derivative at a point;



Simplifying Radical Expressions



Solved Let F X Y Square Root X 2 Y 2 And C 0 0 Chegg Com
Subtracting x^ {2} from itself leaves 0 Subtracting x 2 from itself leaves 0 \left (y\sqrt 3 {x}\right)^ {2}=1x^ {2} ( y 3 x ) 2 = 1 − x 2 Take the square root of both sides of the equation Take the square root of both sides of the equation y\sqrt 3 {x}=\sqrt {1x^ {2}} y\sqrt 3 {x}=\sqrt {1x^ {2}} · In mathematics, a square root of a number x is a number y such that y 2 = x;% Your Function darova on 7 Oct 19 × Direct link to this comment


What Is The Domain Of The Function F X 1 Root X 2 4 Quora



If X 1 Y Y 1 X 0 Then Prove That 1 X 2 Dydx 1 0
\begin{array}{l} \mathbf{if}\;x \leq \cdot 10^{118}\\ \;\;\;\;x\\ \mathbf{elif}\;x \leq \cdot 10^{57}\\ \;\;\;\;\sqrt{{xDerivative at a point; · f (x,y) = \sqrt {x^2y^2} f (x,y)= x2y2 f x ( x, y) = 2 x 2 ⋅ x 2 y 2 f_x (x,y) = \dfrac {2x} { 2 \cdot \sqrt {x^2y^2} } f x (x,y)= 2⋅ x2 y2 2x
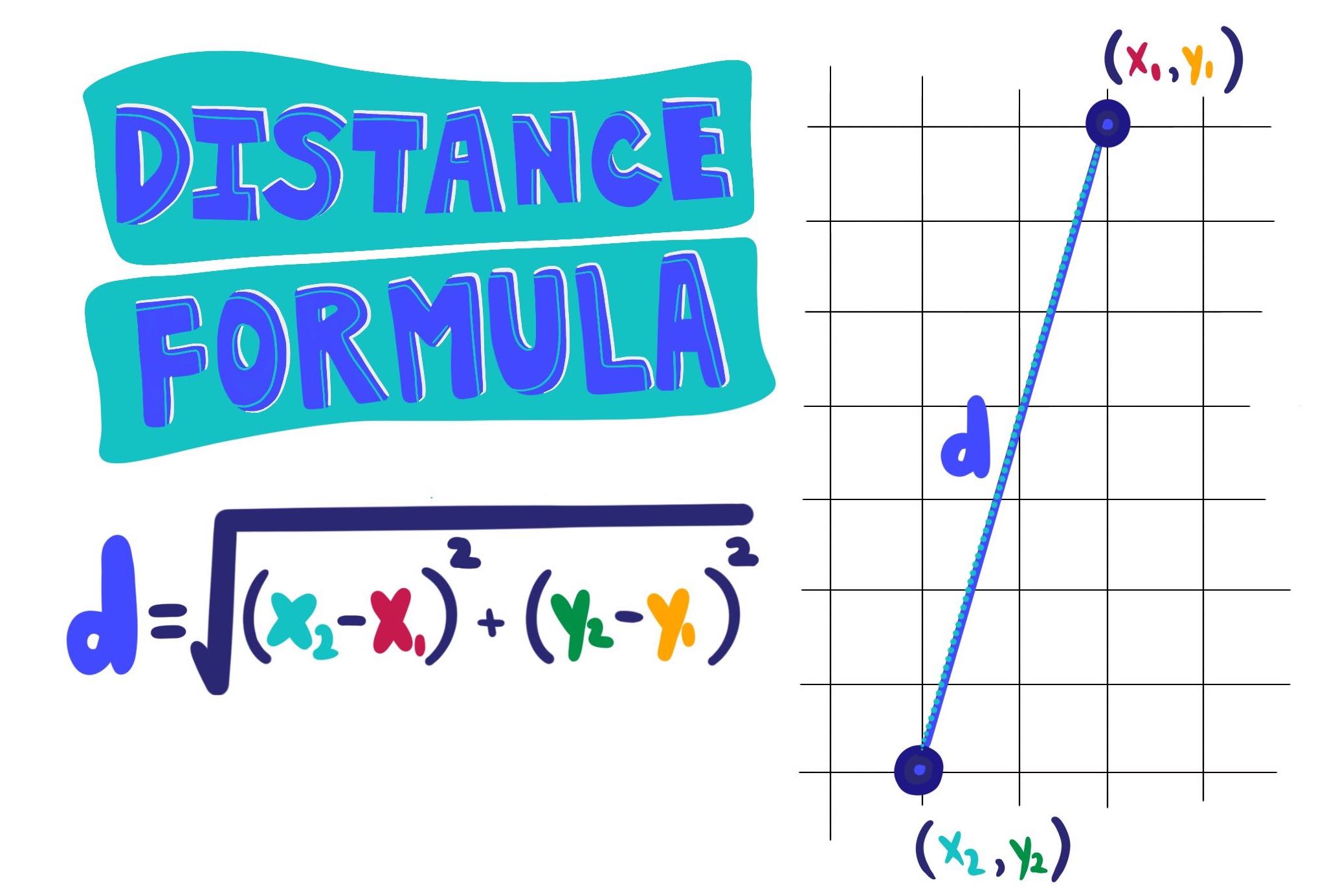


Distance Formula Expii



If X Y In R Satisfy The Equation X 2 Y 2 4x 2y 5 0 Th
Assuming both f and square root are being defined for either ℝ>ℝ or for ℂ > ℂ then the domain is all possible numbers of the set If you start out with ℝ then any real number squared is a positive number, and a square root function is defined fo · Explanation We have to evaluate f (αx,αy) = (αx)(αy) √(αx)2 (αy)2 f (αx,αy) = α2xy √α2(x2 y2) f (αx,αy) = α2xy α√x2 y2 f (αx,αy) = αxy √x2 y2Hence we can define ˜f(x, y) = {f(x, y), (x, y) ≠ (0, 0) 0, x = y = 0 Notice that ˜f(x, y) is continuous on ˉD, which is compact, so ˜f(x, y) is uniformly continuos on ˉD and as a consequence on D It remains to note that ˜f(x, y) ≡ f(x, y) on D Share



Finding Inverse Functions Quadratic Example 2 Video Khan Academy



Sqrt 1 X 2 Y 2 X 2y 2 X Y Dy Dx 0
In other words, a number y whose square (the result of multiplying the number by itself, or y ⋅ y) is x For example, 4 and −4 are square roots of 16, because 4 2 = (−4) 2 = 16Every nonnegative real number x has a unique nonnegative square root, called the principal square root, which is denoted by , · Falls ja, so ist f auch in (0,0) in jede beliebige Richtung und damit total differenzierbar (Die Ableitung ist in jede beliebige Richtung = 0 ) und ich habe die totale Differenzierbarkeit gezeigt Falls dies soweit stimmt bleibt also noch zu zeigen, dass f im Nullpunkt nicht stetig differenzierbar ist \blue Reicht es aus hierzu zu sagen, dass die partiellen Ableitungen in x_0 = (0,0) nicht```(dz)/(dx) = 1/sqrt(x^2 y^2) (d/(dx) sqrt(x^2 y^2))` since `d/(dx) ln(f(x)) = (f'(x))/f(x)` `d/(dx) sqrt(x^2 y^2) = (1/2)(x^2y^2)^(1/2)(2x) = x/sqrt(x^2y^2)`
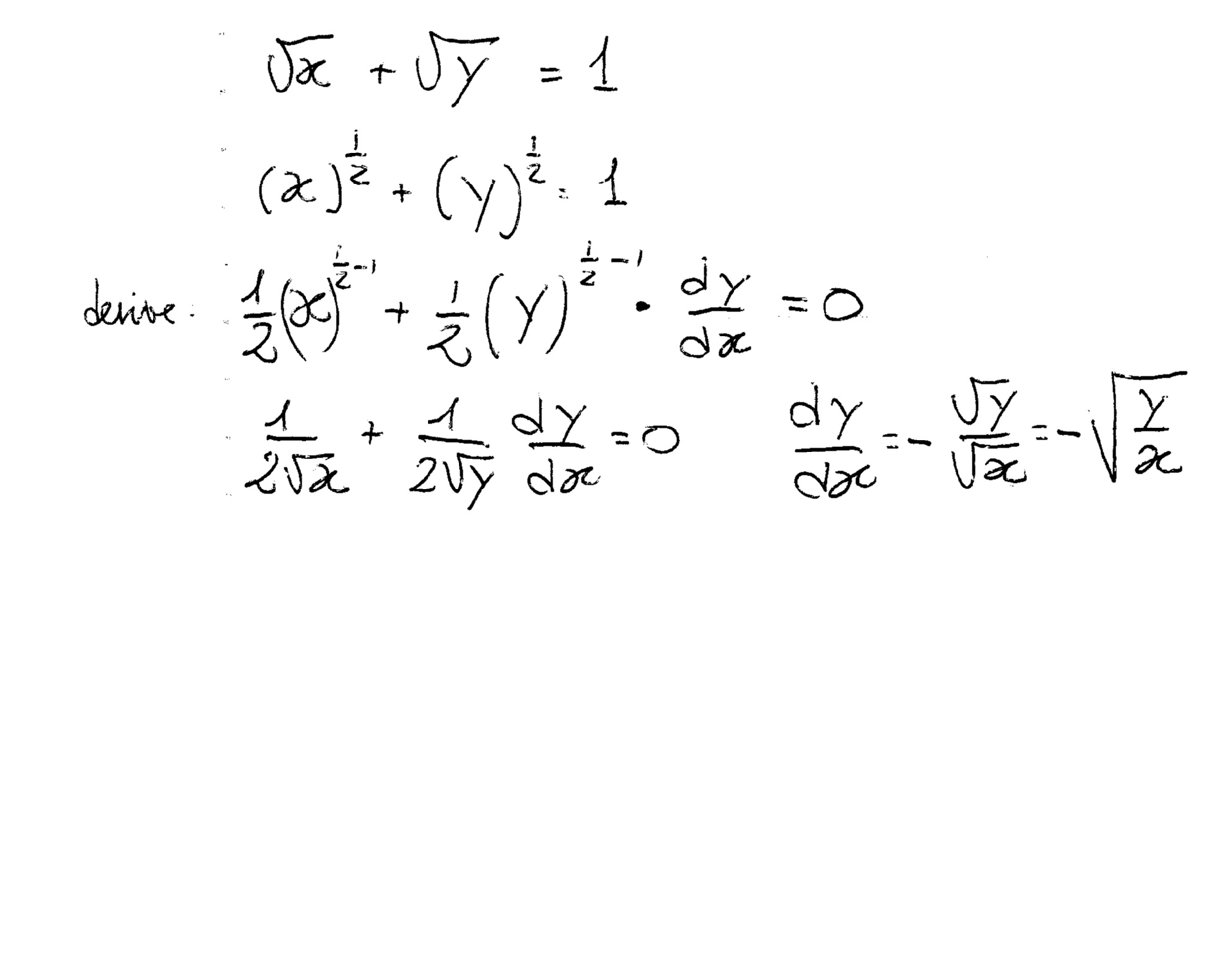


How To Find The Derivative Of Sqrt X Sqrt Y 1 By Using The Implicit Differentiation Socratic



Find The Domain And Range Of The Function F X 1 Sqrt X 5
F(x,y) = sqrt (81x^2y^2) Find the partial derivative of f with respect to x of the function f(x,y) = xye^(x3)y check_circle Expert Answer Want to see the stepbystep answer?1418 · f(x,y)=x^3/(x^2y^2) Stetig differenzierbar Meine Frage Hallo, ich konnte leider an dem wo Differenzierbarkeit im R^n angefangen wurde nicht zur Uni und wollte mich deshalb zum einen vergewissern, ob ich die Herangehensweise richtig verstanden habe und zum anderen wollte ich wissen, wie man mit der Stetigkeit bei der Aufgabe weiter kommt Also die AufgabeStack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange
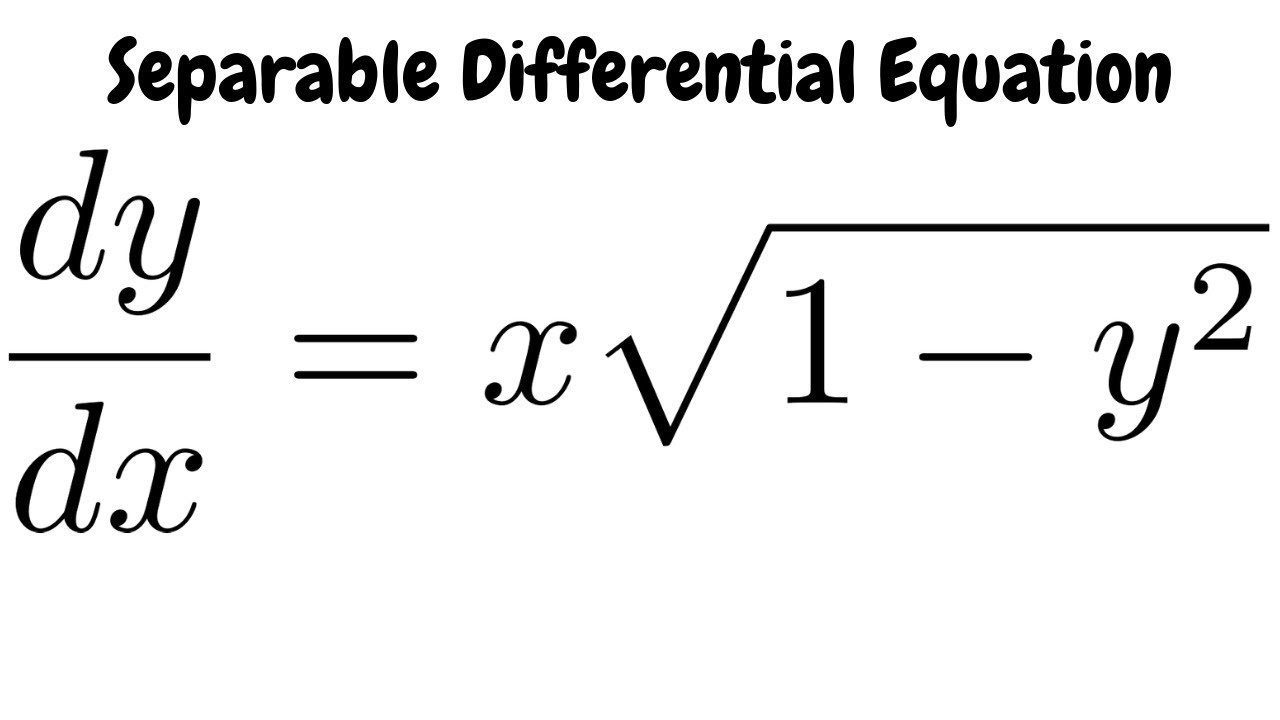


Separable Differential Equation Dy Dx X Sqrt 1 Y 2 Youtube


Draw The Graph Of Y Sqrt X 2 2
0313 · Homework Statement Find the limit, if it exists, or show that the limit does not exist Stewarts Calculus 7th edition 142 Q13 Homework Equations The Attempt at a Solution f(x,y)=\\frac{xy}{\\sqrt{x^{2}y^{2}}} The limit along any line through (0,0) isO domínio de \(f\) é o disco unitário fechado \(x^2y^2\leq 1\) Para todo ponto \((x_0,y_0)\) na fronteira do disco, temos \ \lim_{(x,y)\to(x_0,y_0)}\sqrt{1x^2y^2} = \sqrt{1x_0^2y_0^2} = 0\ Como o mesmo vale também para pontos interiores ao disco, temos que \(f\) éCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music WolframAlpha brings expertlevel knowledge and



Volume Of Region Bounded By Z 4 Sqrt X 2 Y 2 And Z Sqrt X 2 Y 2 Mathematics Stack Exchange
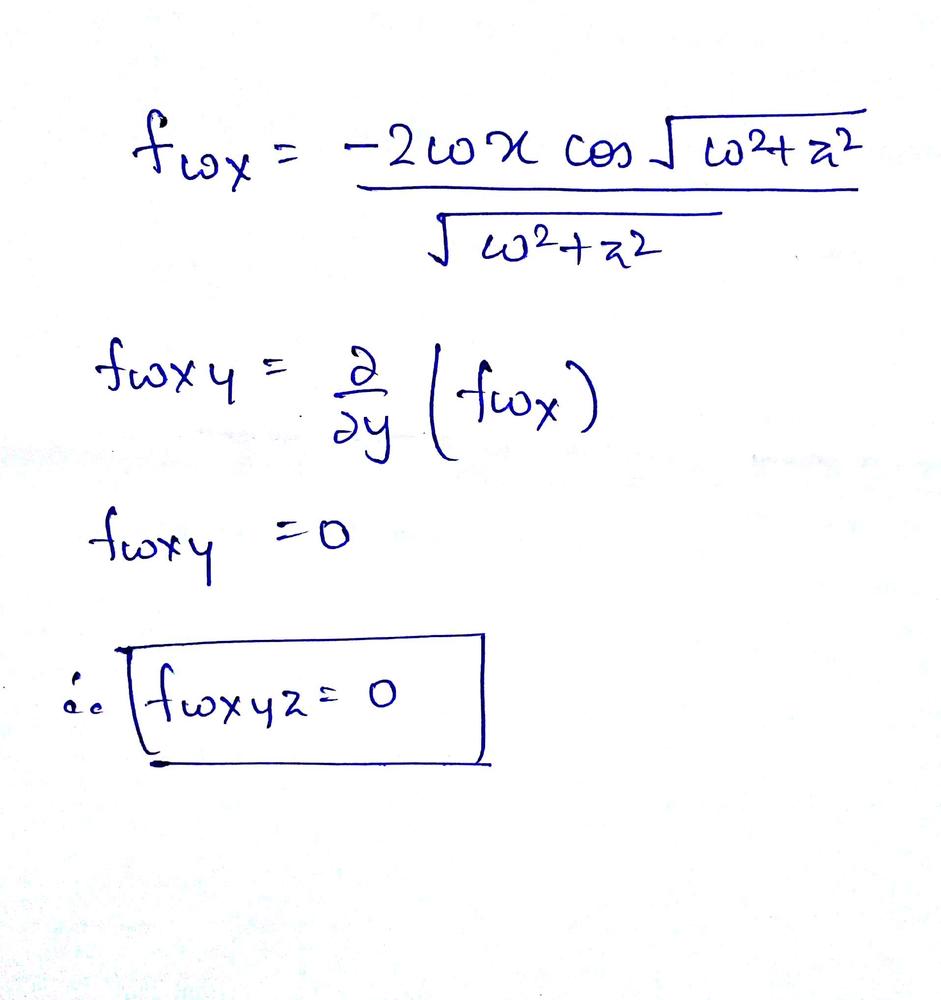


Find The Indicated Partial Derivatives Math F W X Y Z Sqrt X Y Y Z X 3 Sin Sqrt W 2 Z 2 F X X F Y Y F W X Y Z Math Homework Help And Answers Slader
F (x,y) = Sqrt (y) Sqrt (25x^2y^2) Find And Sketch The Domain Of The FunctionWenn Sie bei der GoogleSuche die Formel '12(sqrt(1(sqrt(x^2y^2))^2) 1 x^2y^2) * (sin (10 * (x*3y/57))1/4) from 16 to 16' eingeben, kommt dieseAbleitung 1/sqrt(x^2y^2) dx im MatheForum für Schüler und Studenten Antworten nach dem Prinzip Hilfe zur Selbsthilfe Jetzt Deine Frage im Forum stellen!



Find The Range Of The Following Function Y Sqrt X 2 4 Youtube



How Do You Find The Volume Of The Region Bounded By Y Sqrt X Y 0 X 0 And X 4 Is Revolved About The X Axis Socratic
Als deren Umkehrfunktionen bilden Arkussinus und Arkuskosinus einen Wert aus , wieder auf einen · Average value of f(x,y,z) = sqrt(xyz) over a solid z=4x^2 & x^2y^2=4 & xyplane Thread starter VinnyCee;Gradient sqrt(x^2y^2), \at(2,2) Derivatives First Derivative;
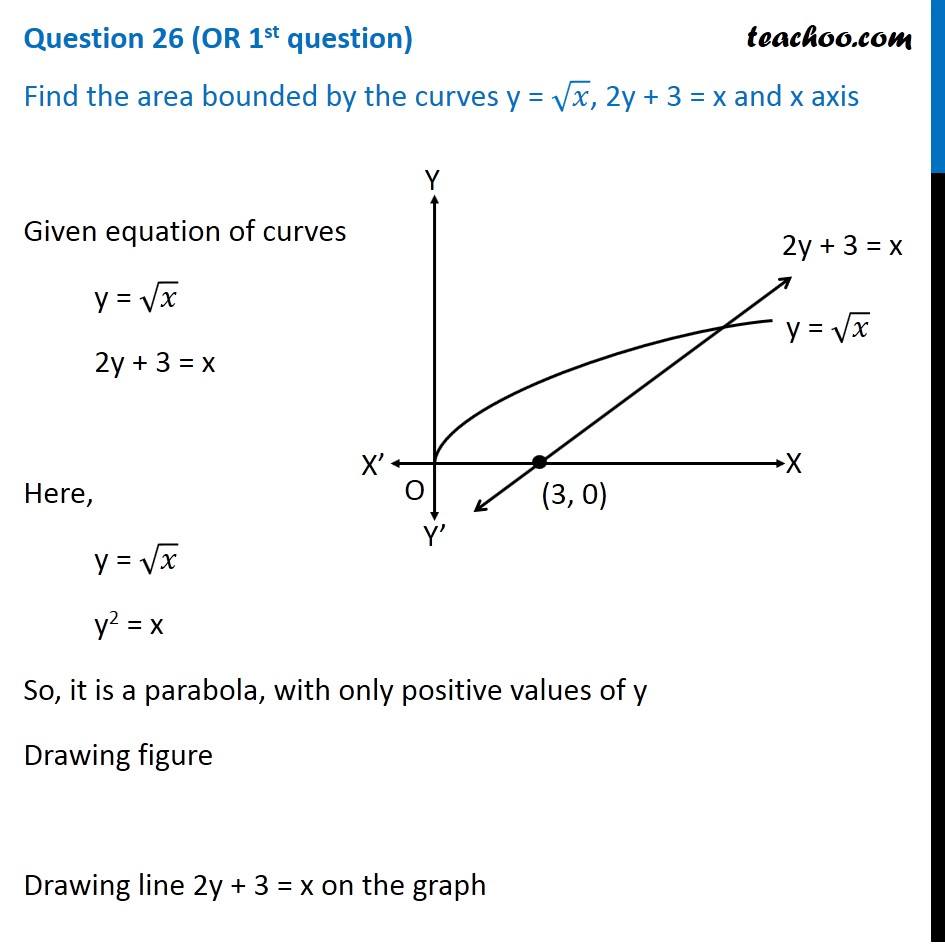


Find The Area Bounded By Curves Y X 2y 3 X And X Axis



Volume With Cross Sections Perpendicular To Y Axis Video Khan Academy
Second Implicit Derivative ; · \\begin{align} 1 \leq y \leq y \\ 0 \leq x \leq \sqrt{1 y^2} \\x^2 y^2 \leq z \leq \sqrt{x^2 y^2} \end{align}\ The first two inequalities describe the right half of aSketch the graph of f f(x, y)=\sqrt{9x^{2}y^{2}} Vector Basics Example 1 In mathematics, a vector (from the Latin "mover") is a geometric object that has a magnitude (or length) and a
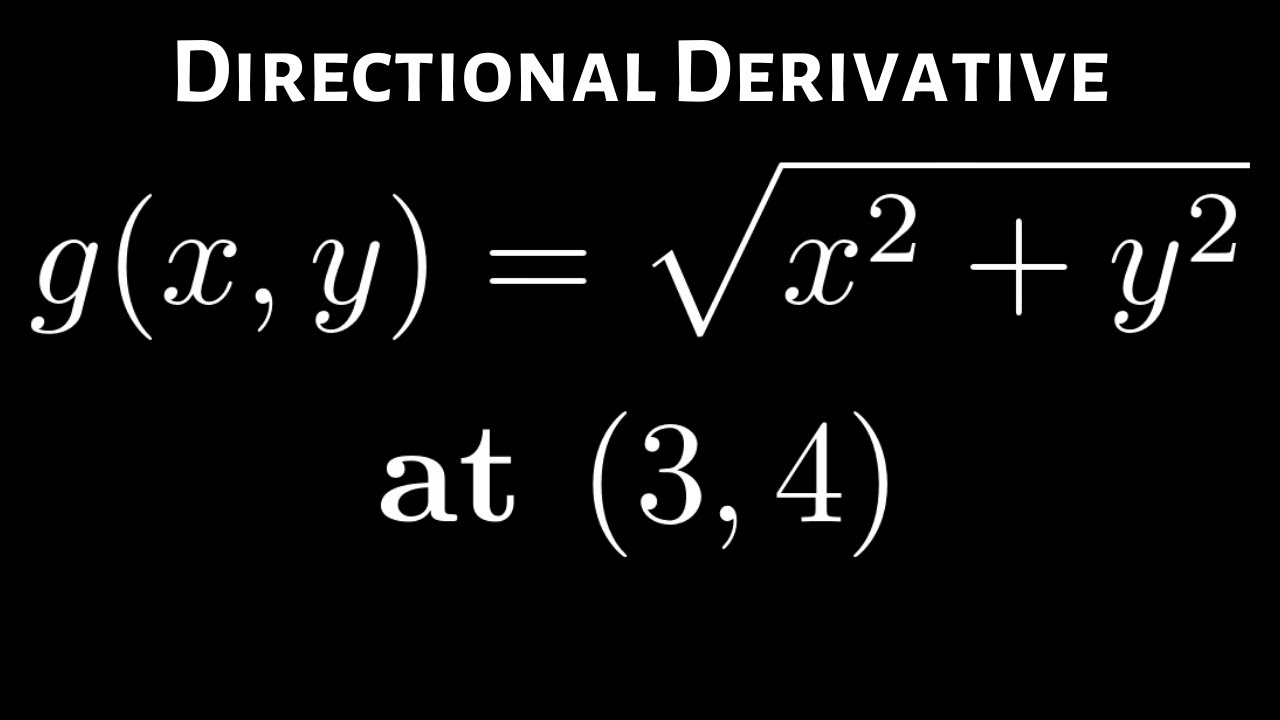


Directional Derivative Of G X Y Sqrt X 2 Y 2 At 3 4 In The Direction Of V 8i 15j Youtube



Implicit And Logarithmic Differentiation
Higher Order Derivatives ;Das Wurzelziehen ist ja die Umkehrung des Quadrierens Die Quadratfunktion lautet $$y = f(x) = x^2$$ Wird der Definitionsbereich der Quadratfunktion $$y = f(x) = x^2$$ auf den Bereich $$x ge 0$$ eingeschränkt, gehört zu jedem yWert genau ein xWert Damit besitzt die Funktion $$f$$ eine Umkehrfunktion $$f^1$$F(x,y,z)=sqrt(25 x 2 y 2 z 2) Can someone explain how I should go about this question?



Evaluate The Double Integral Xysqrt X 2 Y 2 Dydx Without Using Trigonometric Substitutions Youtube



Transforming The Square Root Function Video Khan Academy
· To find the range, notice that sqrt must result in a number that's nonnegative, so the range must be a subset of 0, infinity) Take the points on the x axis, ie points of the form (x, 0) We get f(x, 0) = sqrt(x^2) = x The range of f(x, 0) = x is all 0, infinity), so the range of all f(x, y) is also 0, infinity)Specify Method (new) Chain Rule;Solve the diffrential equation yy' x = sqrt (x^2 y^2 ) at y(3) = 4 Top Answer answer is here ATTACHMENT PREVIEW Download attachment CaptureJPG Sign up to view the full answer View Full Answer Other Answers Solution The complete solution is given into the attach file
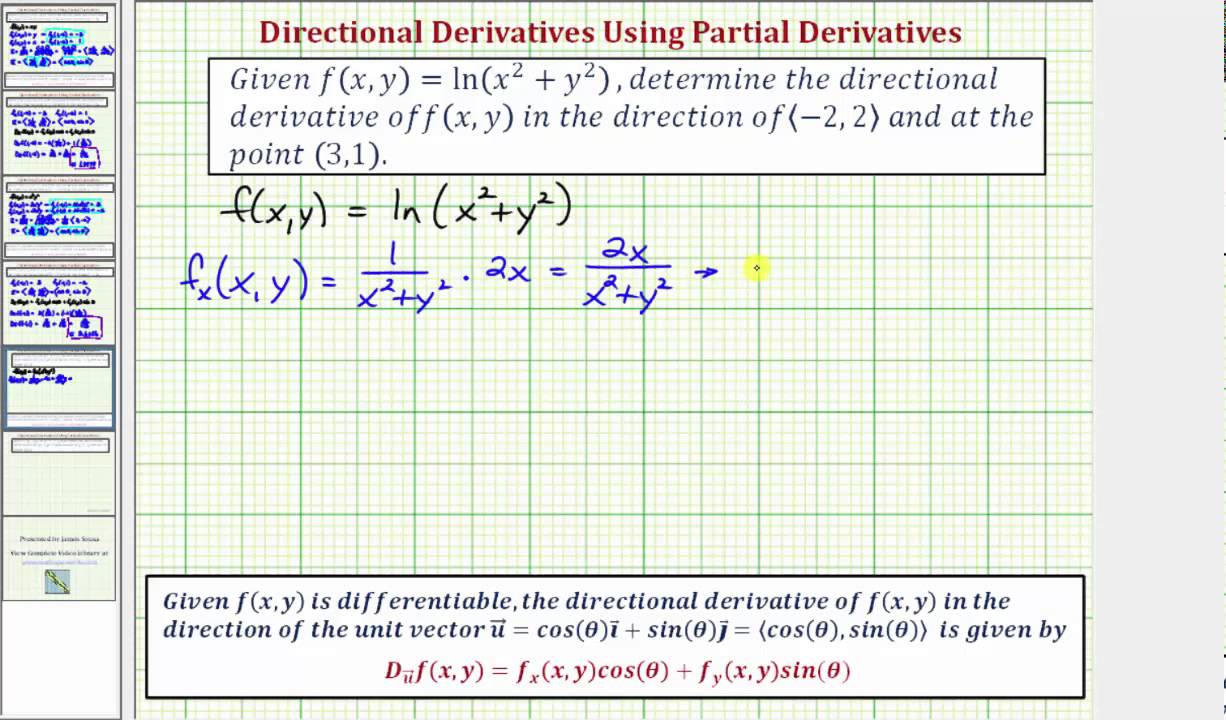


Ex 3 Find A Value Of A Directional Derivative F X Y Ln X 2 Y 2 Youtube



Show That F X Y Sqrt X 2 Y 2 Has One Critical Point P And That F Is
· Σ the surface of your function F F it's a surface of revolution because F (x,y) = f (r) F ( x, y) = f ( r) where r = sqrt (x^2y^2) r = √ x 2 y 2 Precisely, f (r) = sqrt (r^21) ln (4r^2)Sign up with Facebook or Sign up manually Already have an account?Limit as (x,y) approaching (0,0) of (x^2y^2)/(sqrt(x^2y^21)1) Derivatives First Derivative;



22m 28 Spring 05 J Simon An Example Of Computing A Triple



Solved Suppose That F Is An Inverse Square Force Field I Chegg Com
0401 · Aloha ) Die beiden partiellen Ableitungen bekommst du mit der Kettenregel ∂ ∂ x 4 − x 2 − y 2 = 1 2 4 − x 2 − y 2 ⋅ ( − 2 x) = − x 4 − x 2 − y 2 \frac {\partial} {\partial x}\sqrt {4x^2y^2}=\frac {1} {2\sqrt {4x^2y^2}}\cdot (2x)=\frac {x} {\sqrt {4x^2y^2}} ∂x∂Start date Apr 14, 05;We will derive a contradiction Suppose that \frac {x^2} {\sqrt {x^2y^2}}=f (x)g (y) for some functions f and g Then f (1)g (1)=\frac {1} {\sqrt {2}}, It cannot be done Suppose to the contrary that it can be done We will derive a contradiction Suppose that x2y2
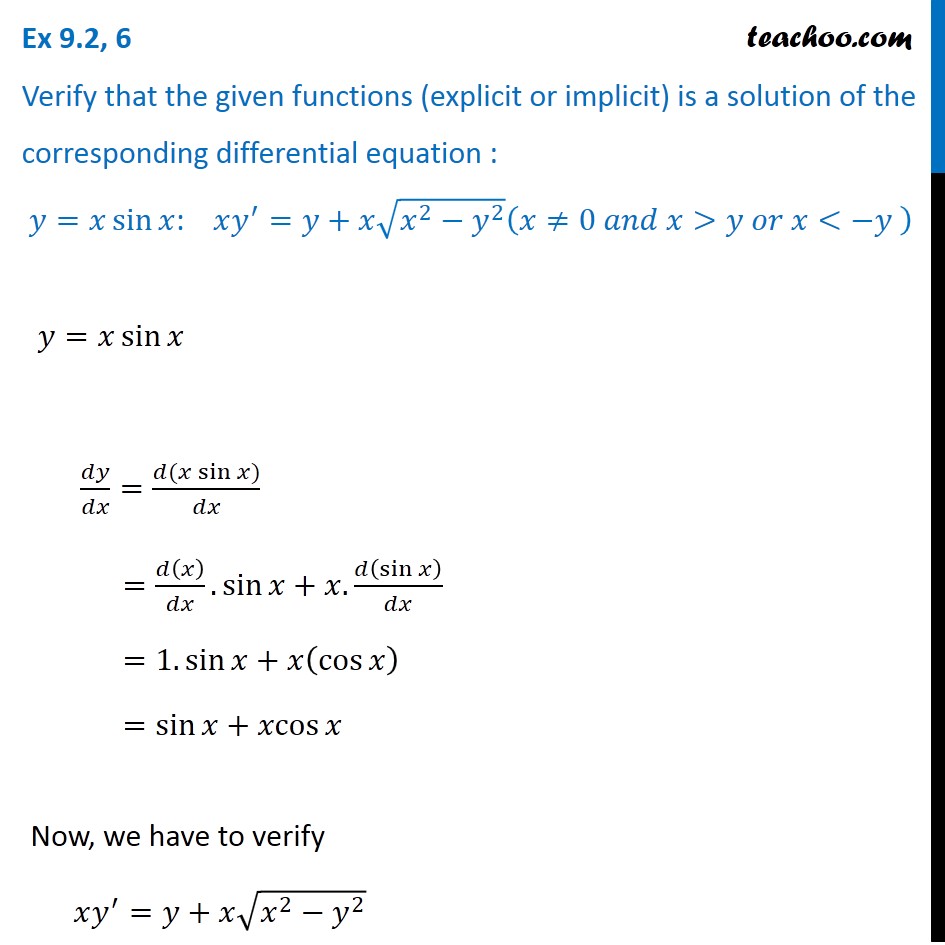


Ex 9 2 6 Verify Y X Sin X Xy Y X Root X2 Y2



Using Integration Find Area Of Region X Y X 2 Y 2 1 X Y
See the answer graph f(x y)=sqrt(9x^2y^2) Expert Answer 100% (1 rating) Previous question Next question Get more help from Chegg Solve it with our calculus problem solver and calculatorFirst, note that the domain of the function is given by math 4 x^2 y^2 \geq 0 /math, or math x^2 y^2 \leq 4 /math, which is a disk of radius 2 centered at the origin in the xyplane Also, note that if we let math z = f(x,y) /mathPergunta Calcular as funções derivadas parciais de Z=f(x,y)=rais de 9x^2y^2 Em seguida, calcular fx (2,1) e fy(2,1) enviada por Jose J R Junior para UNIDERP



Introduction To Matlab
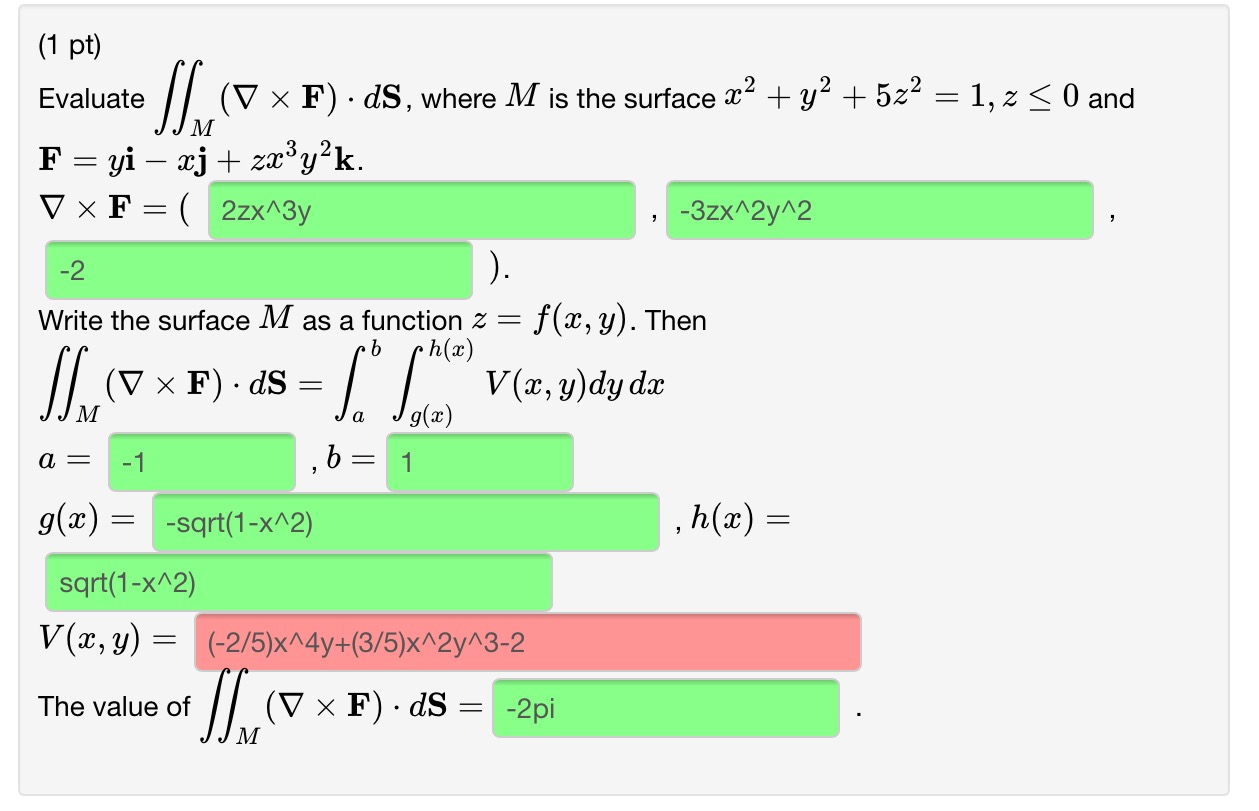


Solved I Really Need Help Finding V X Y The Rest Is Corr Chegg Com
· a f(x,y)=2 bf(x,y)=3 cf(x,y)=4 also d Find k such that the level surface f(x,y)=k consist of a single point Answer For a,b,c the equation for a level curve is F(x,y)=k Therefore the answers are 1/sqrt(1x^2y^2) = 2, 1/sqrt(1x^2y^2) = 3, 1/sqrt(1x^2y^2) = 4, respectfully Part D I had a harder time The gradient fcn of x is x/sqrt(1x^2y^2 )^3 and the gradient fcn of y is y/sqrtUsing the values calculated above, the gradient vector of the given scalar function will be ∇f (x,y) = ∂ ∂x, ∂ ∂y = 12xy 2y√xx2y, −x(2xy) 2y2√xx2y ∇ f ( x, y) = ∂ ∂ x
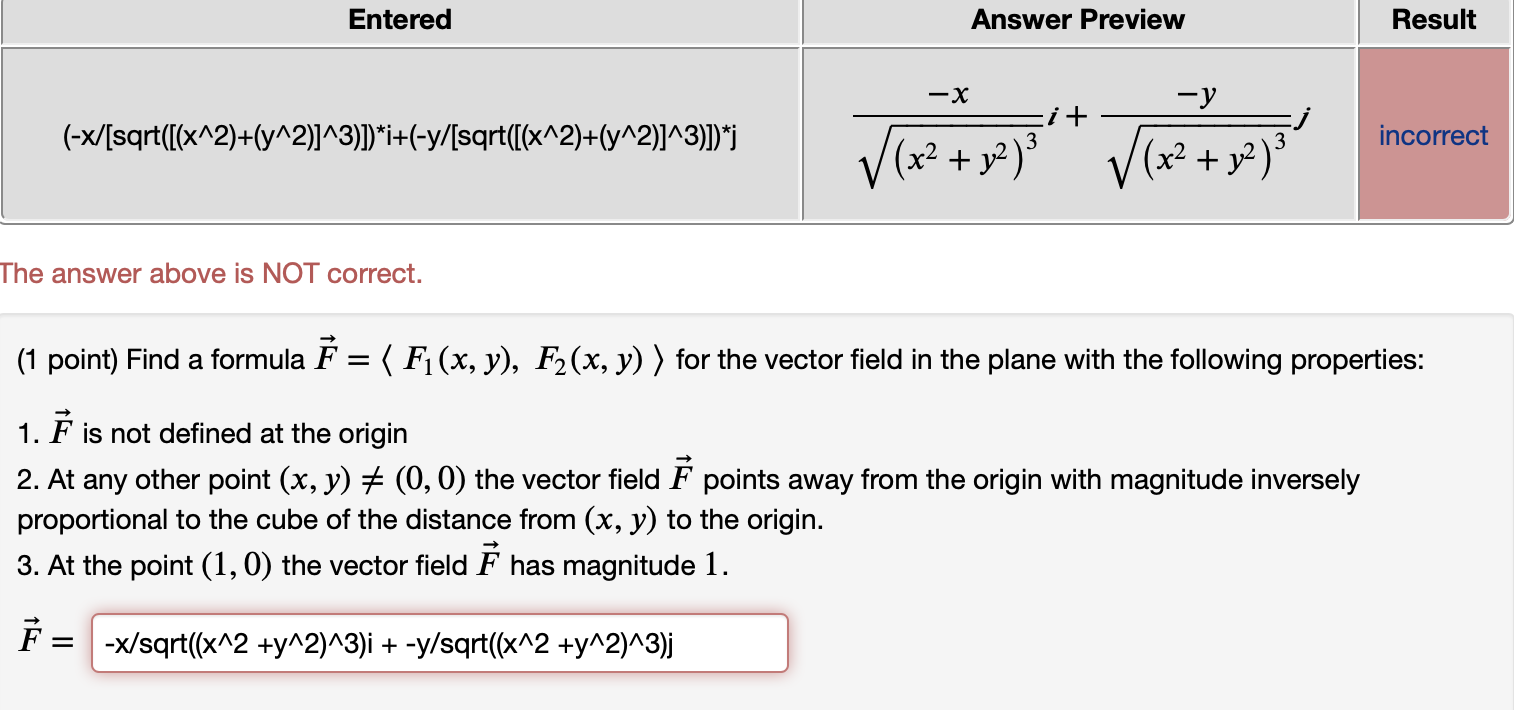


Solved Entered Answer Preview Result X V It X Sqrt Chegg Com



Solved Consider The Function F That Is Given By F X Y Chegg Com



Prove That F X Sqrt X Is Uniformly Continuous On 0 Infty Mathematics Stack Exchange


How Do You Sketch The Region Enclosed By The Given Curves And Decide Whether To Integrate With Respect To X Or Y Then Find The Area Of The Region Of 2y 3x



How Can I Prove That Xy Leq X 2 Y 2 Mathematics Stack Exchange
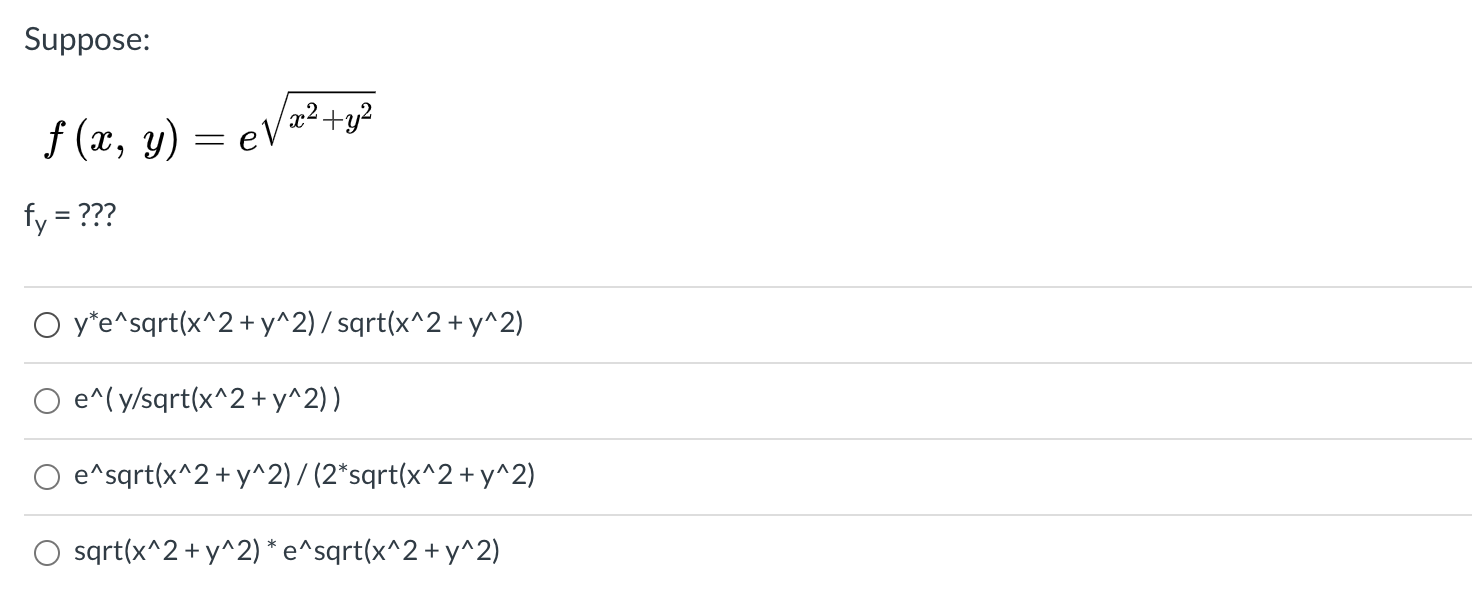


Solved Suppose F X Y Eve E V X Y2 Fy O Y E Chegg Com
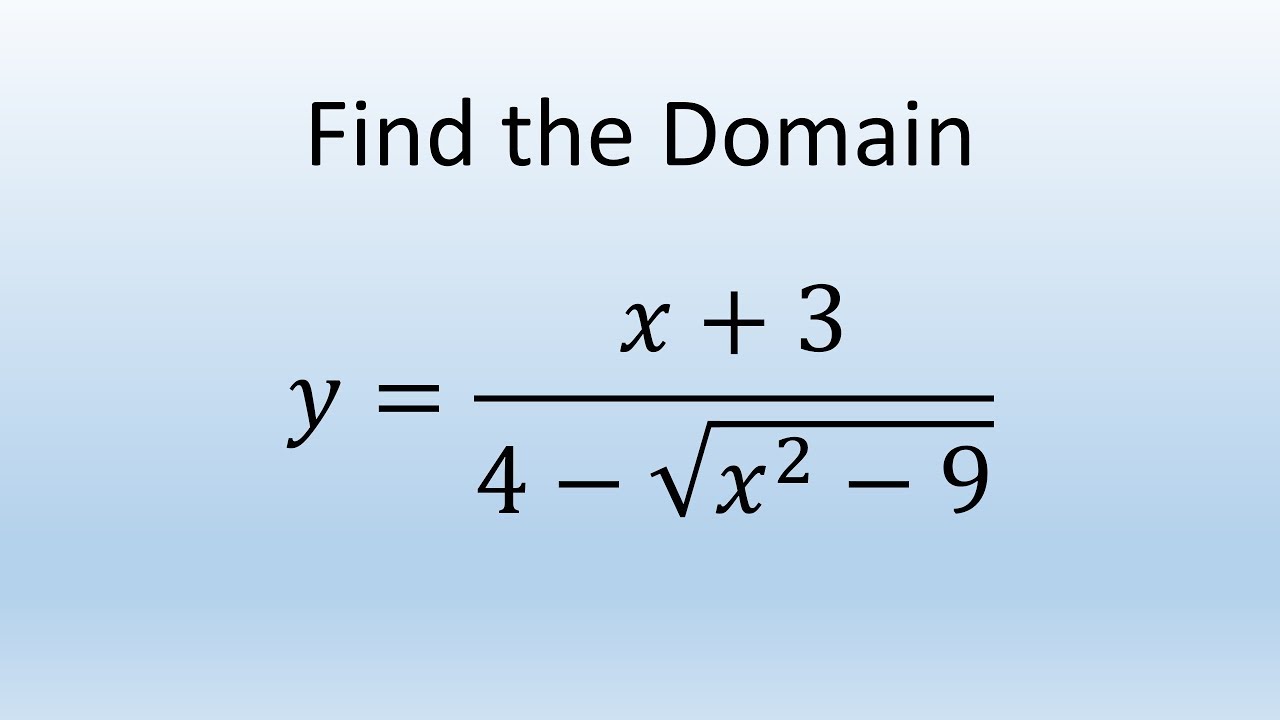


Find Domain Of Function Y X 3 4 Sqrt X 2 9 Youtube
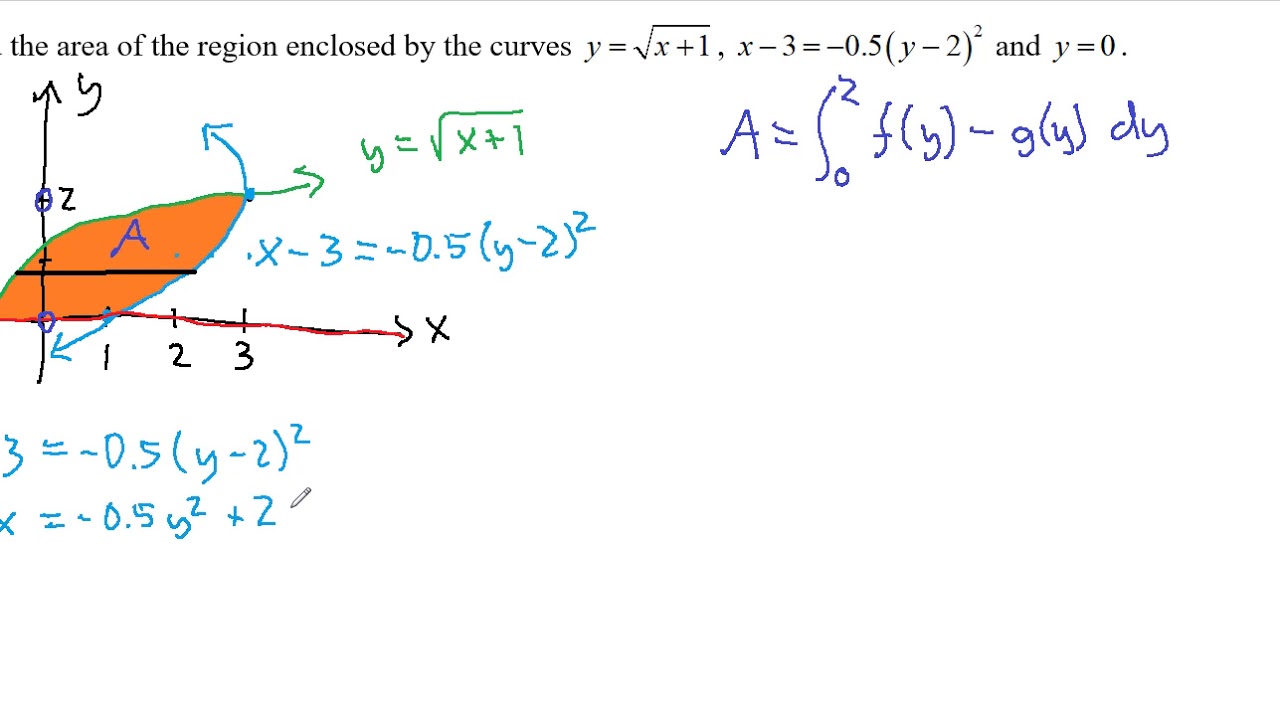


Average Value And Area Revisited
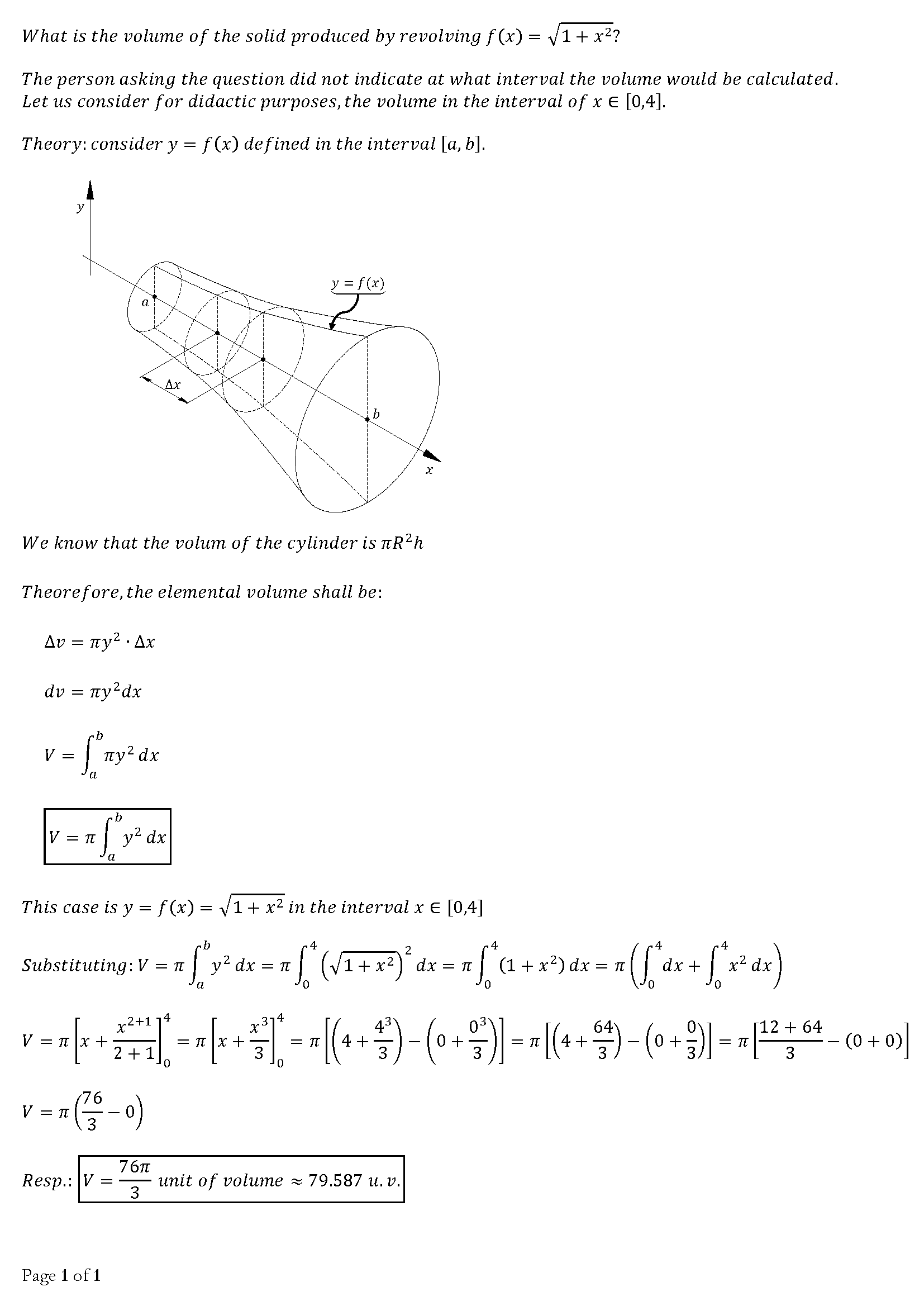


What Is The Volume Of The Solid Produced By Revolving F X Sqrt 1 X 2 Around The X Axis Socratic



Mean Value Theorem Example Square Root Function Video Khan Academy



X Dy Dx Y Sqrt X 2 Y 2 Where Y X B


How To Plot The Graph For The Following Equation Math Sqrt X Sqrt Y 1 Quora



Find The Range Of The Function F X Sqrt X 2 1 My Doubt Is How Can I Find The Range By Writing Brainly In



Inverse Trigonometric Derivatives F X Arcsin Sqrt X Math Videos Maths Exam Calculus



Differentiate The Function F X Sqrt X Ln X Mathematics Stack Exchange



Show That F X Y X 2ye X 2 Y 2 Has Maximum Values At 1 1 2 And Minimum Values At Show Also That F Has Infinitely Many Other Critical Points And D 0 At



Under The Cone Z Sqrt X 2 Y 2 And Above The Disk X 2 Y 2 4 Youtube
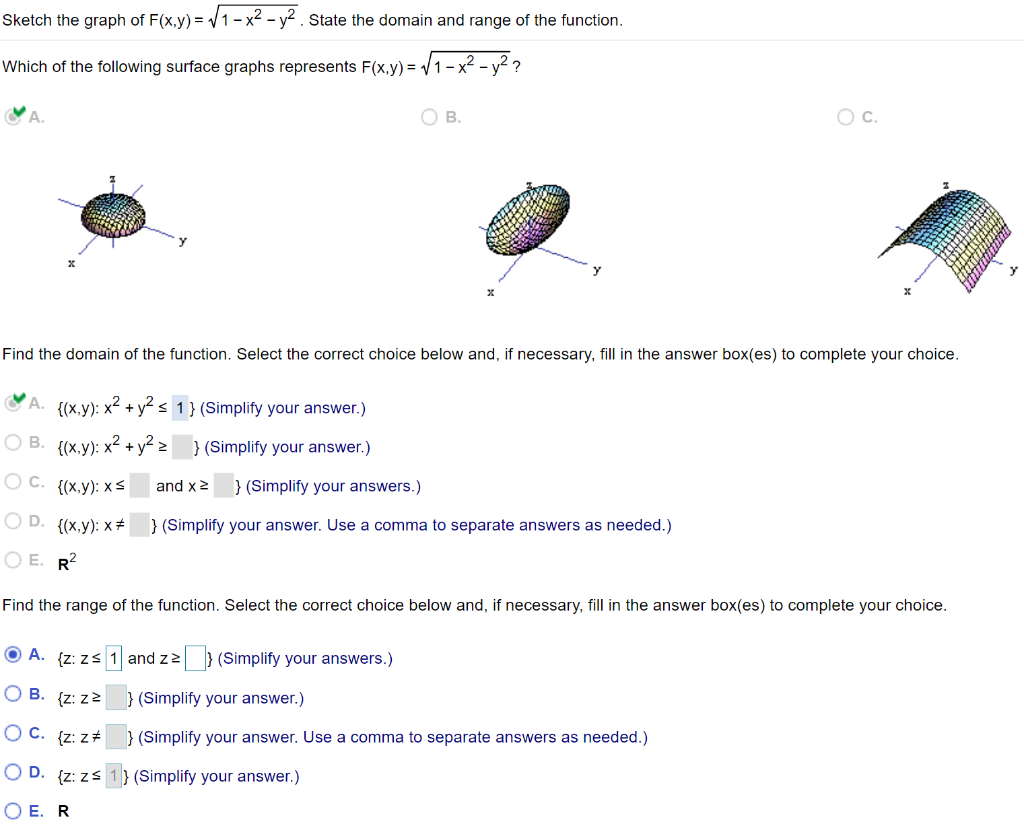


Solved Sketch The Graph Of F X Y Sqrt 1 X 2 Y 2 State Chegg Com



Find The Domain And Range Of Y Sqrt X 2 81


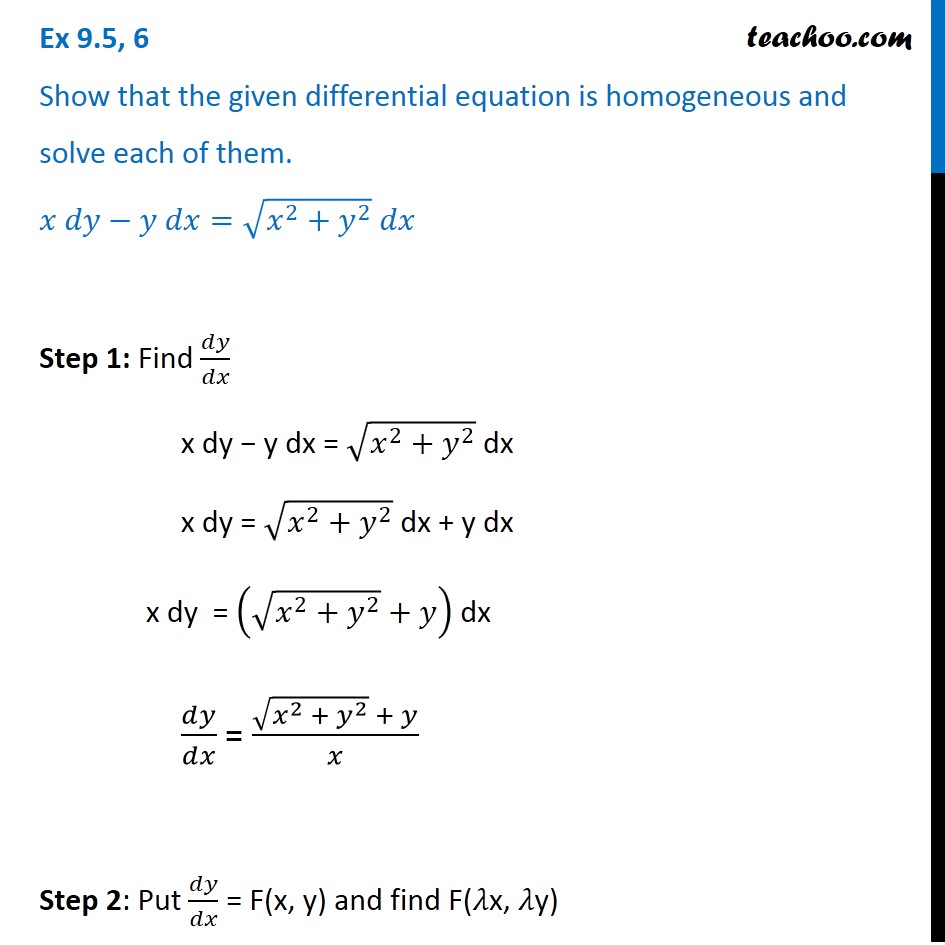
Ex 9 5 6 Solve Differential Equation X Dy Y Dx Root X2 Y2 Dx



Find The Total Differential Of The Multivariate Function F X Y Z E X Maths Exam Math Videos Calculus
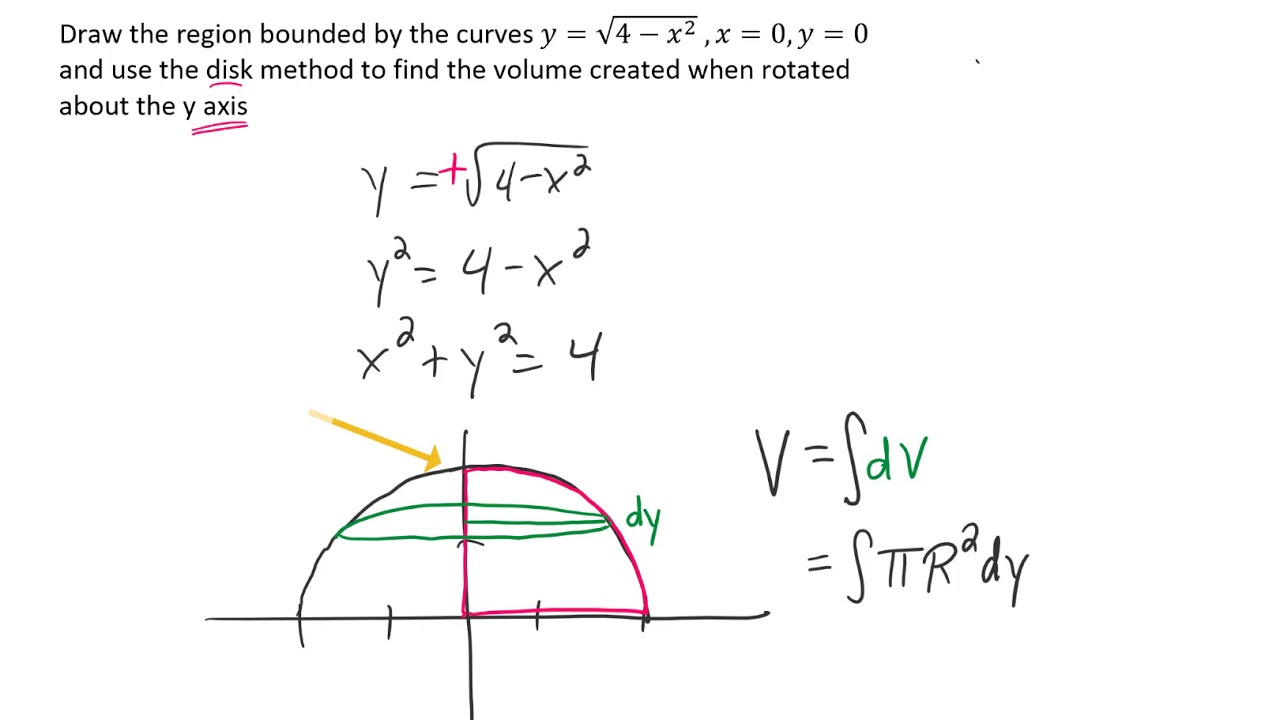


Rotate The Region Bounded By The Curves Y Sqrt 4 X 2 X 0 Y 0 About The Y Axis Find The Volume Youtube
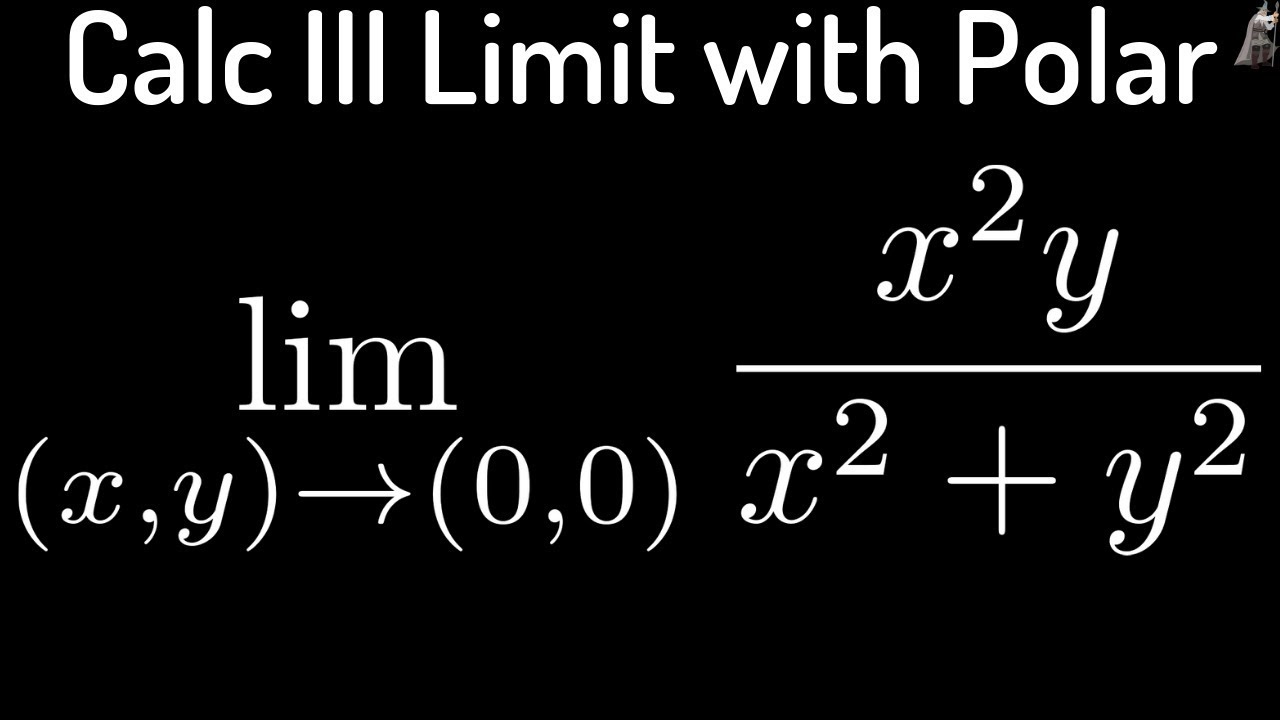


Multivariable Calculus Limit Of X 2y X 2 Y 2 Using Polar Coordinates Youtube



Double Integrals Volume And Average Value
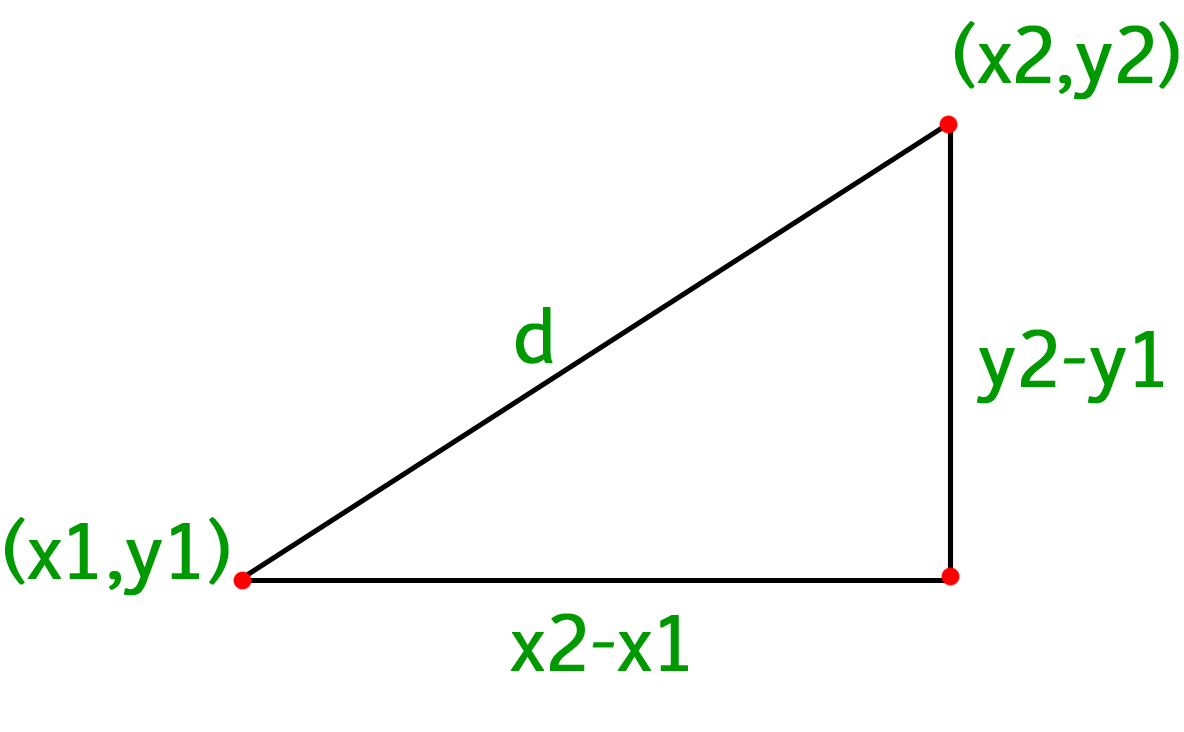


Program To Calculate Distance Between Two Points Geeksforgeeks



The Domain Of The Function F X 1 Sqrt X 2 2 X 8 Is Where



Calc I Linearization Of F X Sqrt X 3 At 1 Youtube
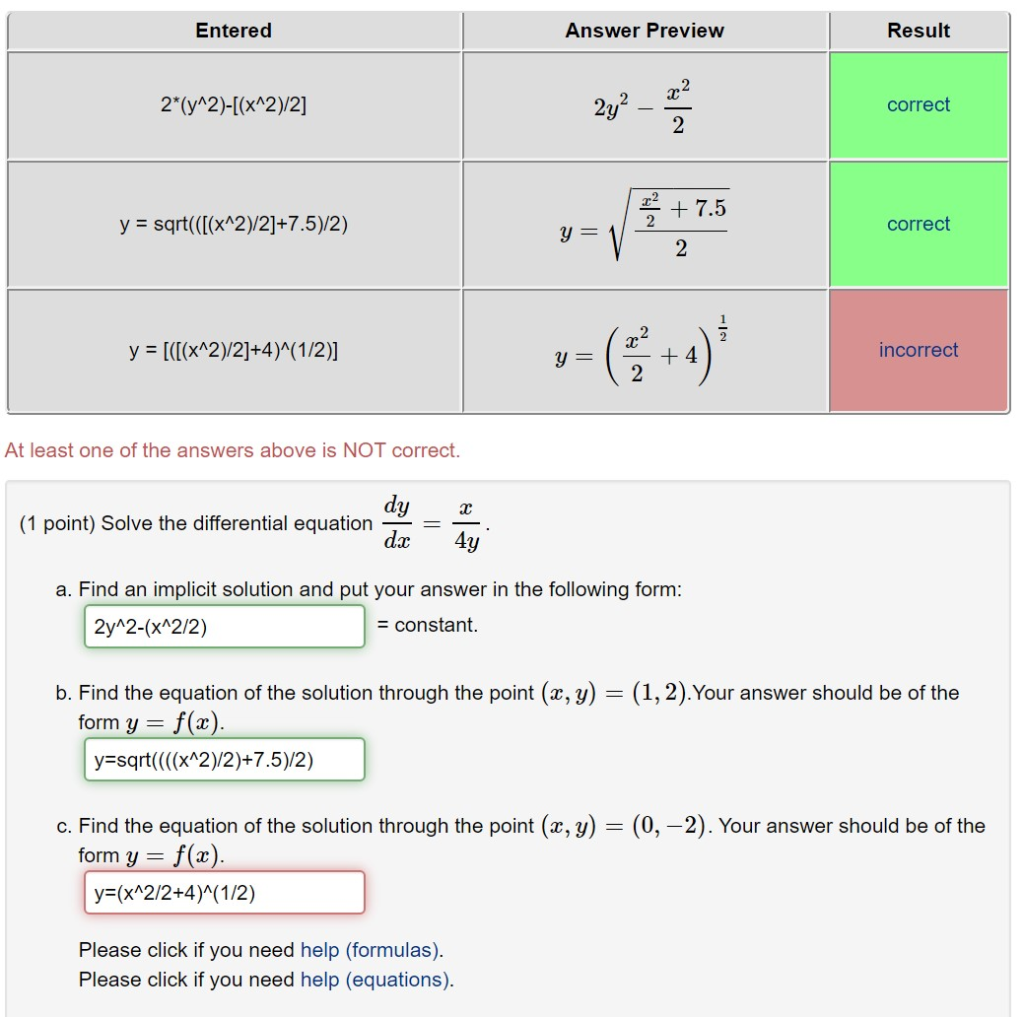


Solved Entered Answer Preview Result 2 Y 2 X 2 2 2o Chegg Com



Limit Of Sqrt X 2 Y 2 Ln Sqrt X 2 Y 2 Using Polar Coordinates And L Hopital S Rule Math Videos Coordinates Calculus



Domain Of F X Y Sqrt X Sqrt Y Youtube



10 11 19 09 14 35 Pi Tangent


Second Order Partial Derivatives F X Y Sqrt X 2 Y 2 Youtube


Solved The Region Is A Cone Z Sqrt X 2 Y 2 Topped By A Chegg Com


Double Integrals Volume And Average Value


Ex 9 5 6 Solve Differential Equation X Dy Y Dx Root X2 Y2 Dx


Horizontal Translation Of Square Root Graphs Expii



If Log X 2 Y 2 Tan 1 Yx Then Prove That Dydx X Yx Y
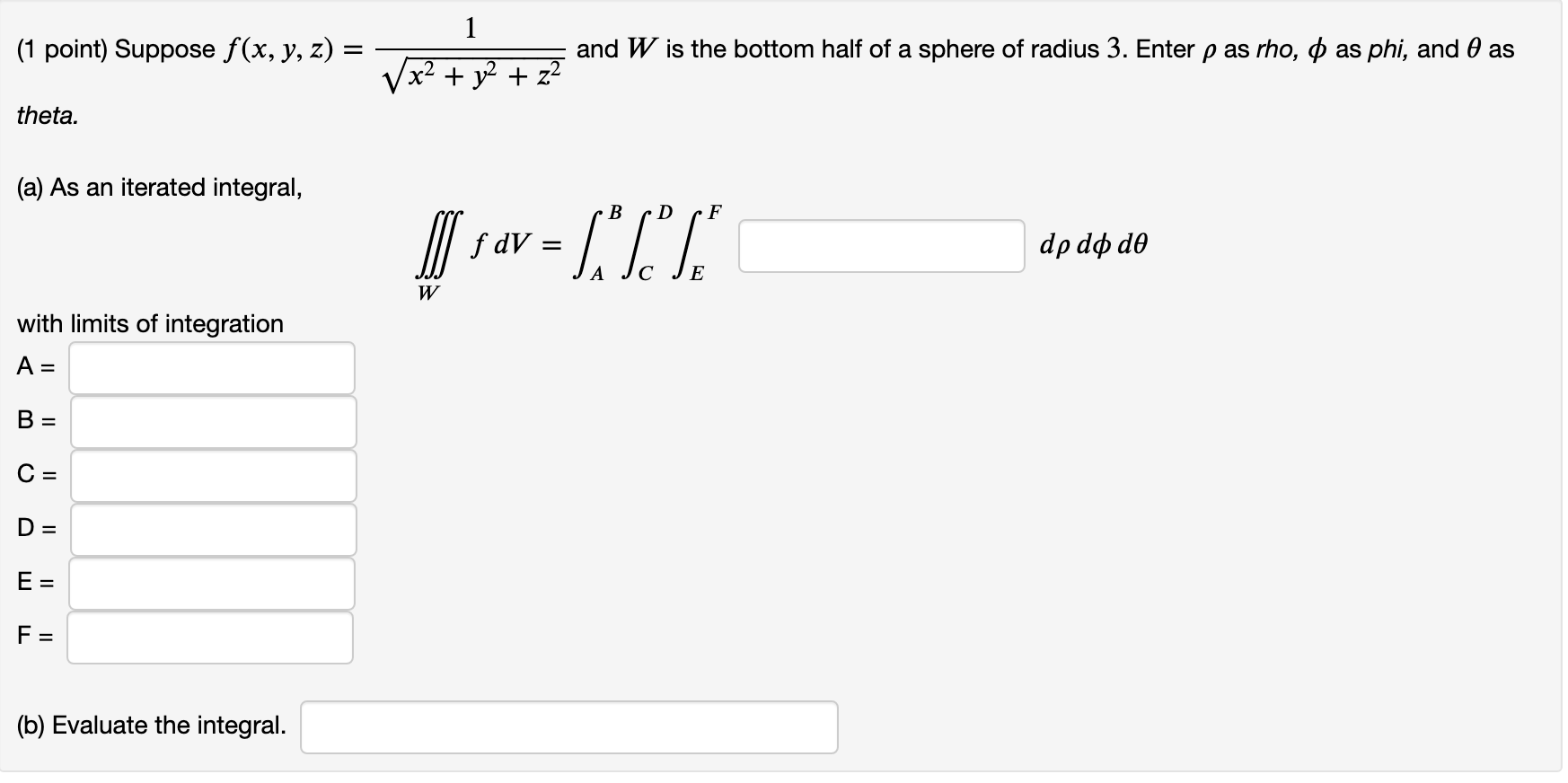


Solved Suppose F X Y Z 1 Sqrt X 2 Y 2 Z 2 And 𝑊w Is The Chegg Com
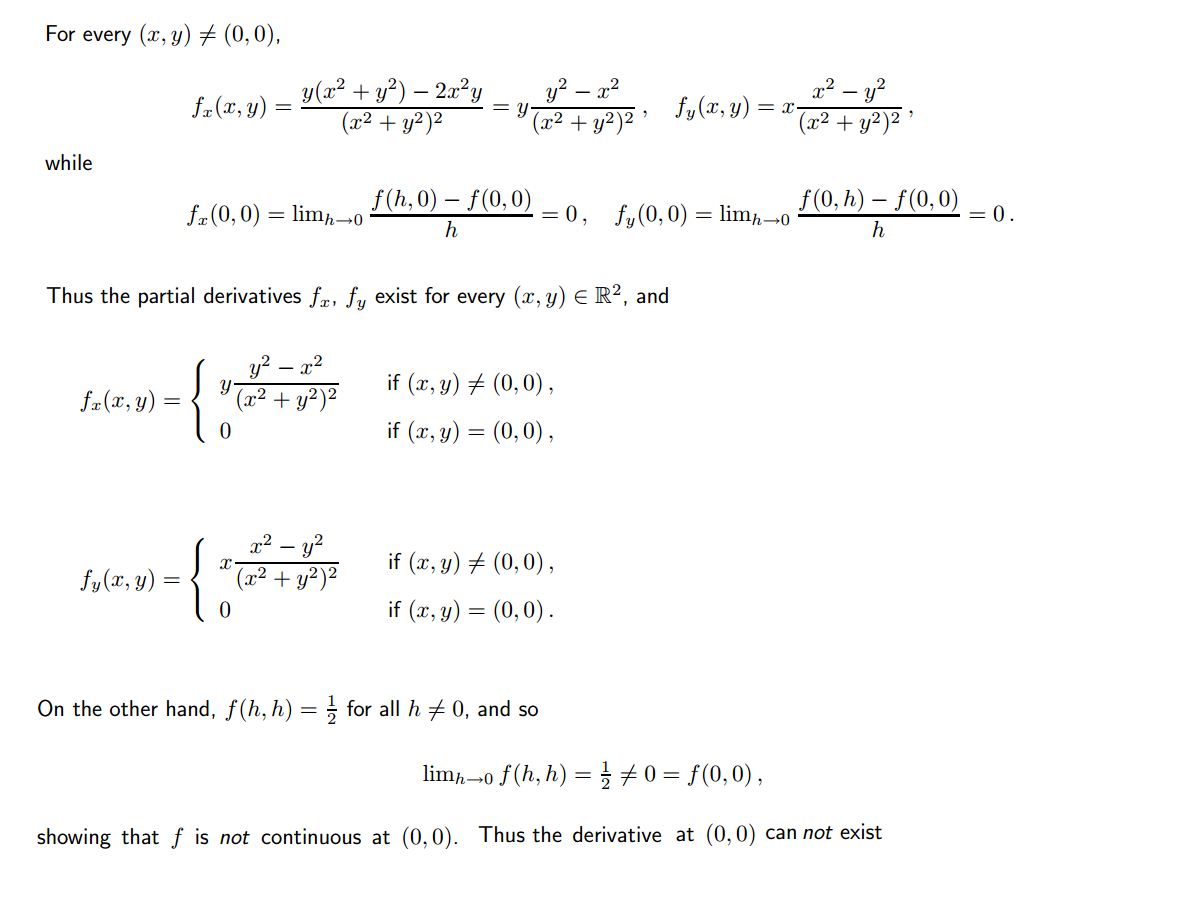


The Function F X Y Xy X 2 Y 2 If X Y Is Not Equal To 0 0 0 If X Y 0 0 Show That Fx 0 0 And Fy 0 0 Both Exist But Is Not Differentiable At 0 0 Homework Help And Answers
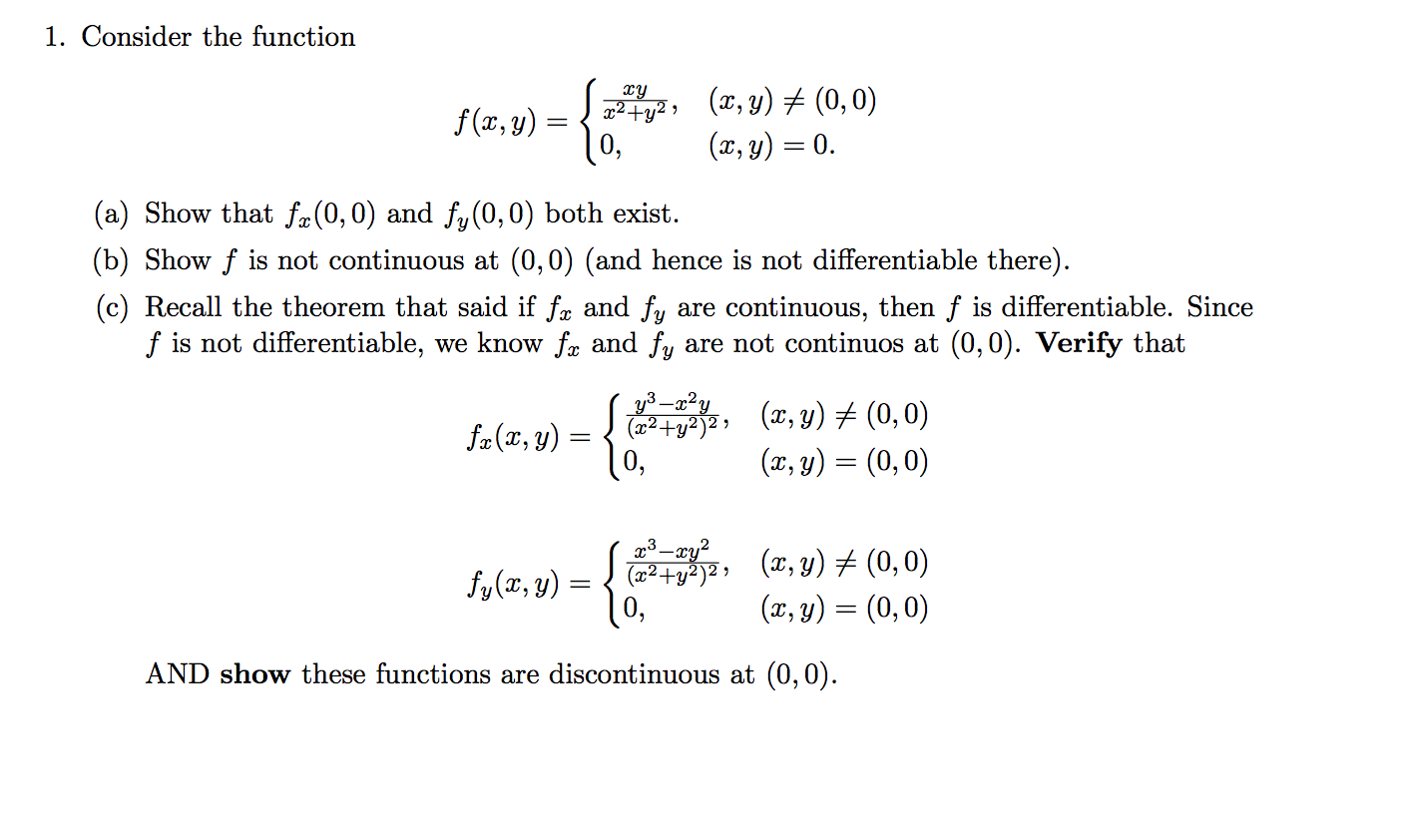


Solved Consider The Function F X Y Xy X 2 Y 2 Chegg Com



Contour Map Of F X Y 1 X 2 Y 2 Youtube
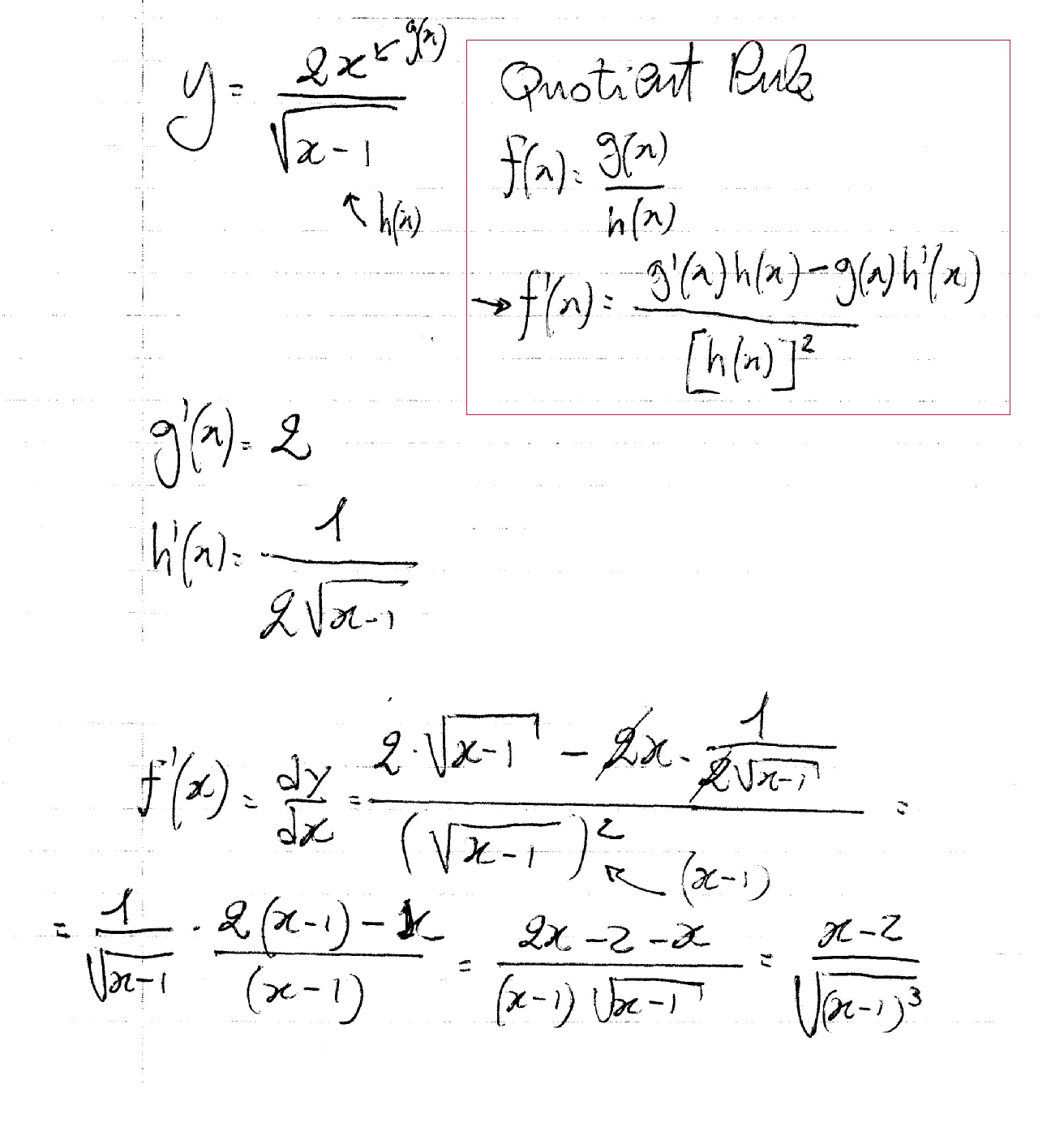


How Do You Find Dy Dx For Y 2x Sqrt X 1 Socratic



Find The Area Of The Region Bounded By The Curves Y Sqrt X Y X 6 And The X Axis By Integral With Respect To X Mathematics Stack Exchange



The Value Of F X Y 4sqrt X 3y 4sqrt X 3 Sqrt Y Sqrt X



Differentiating Related Functions Intro Video Khan Academy



Solutions To Homework Problems On Page 77 V


Horizontal Translation Of Square Root Graphs Expii



Esercizio 3a F Xy Sqrt 3x Y 2 Xy Minipages



How Do You Find The Domain And Range Of Sqrt X 2 4 Socratic
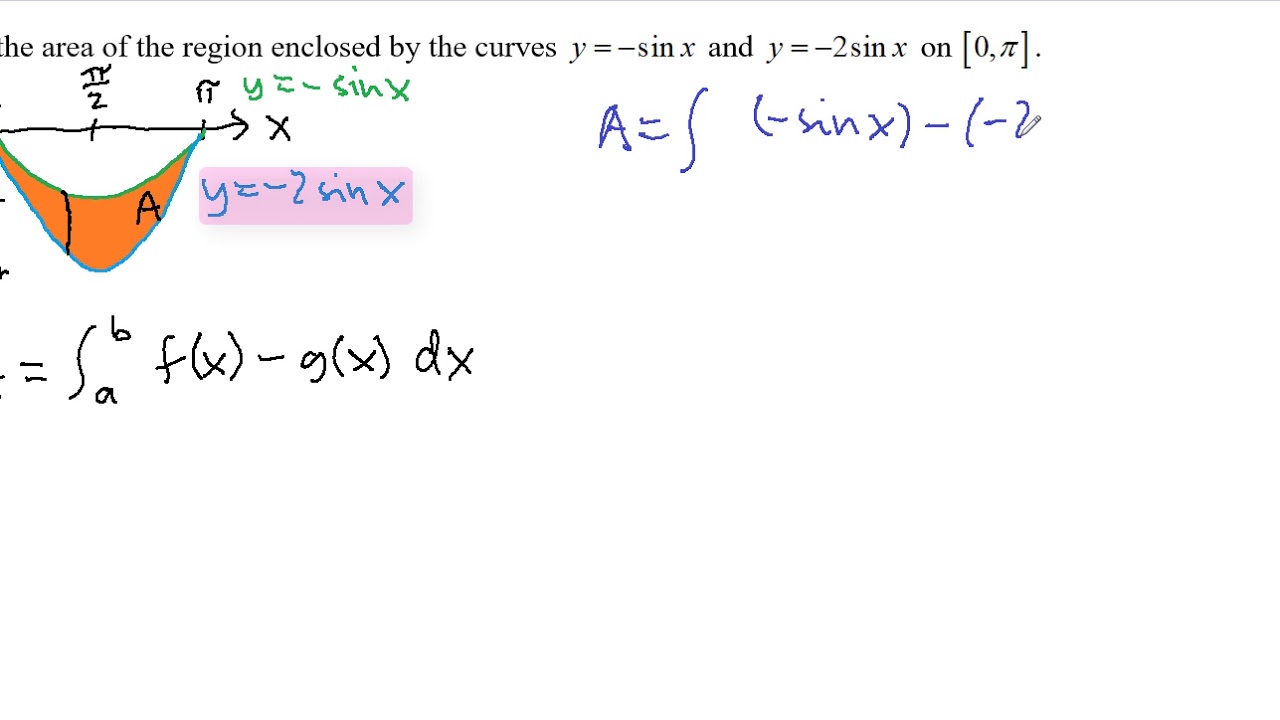


Average Value And Area Revisited
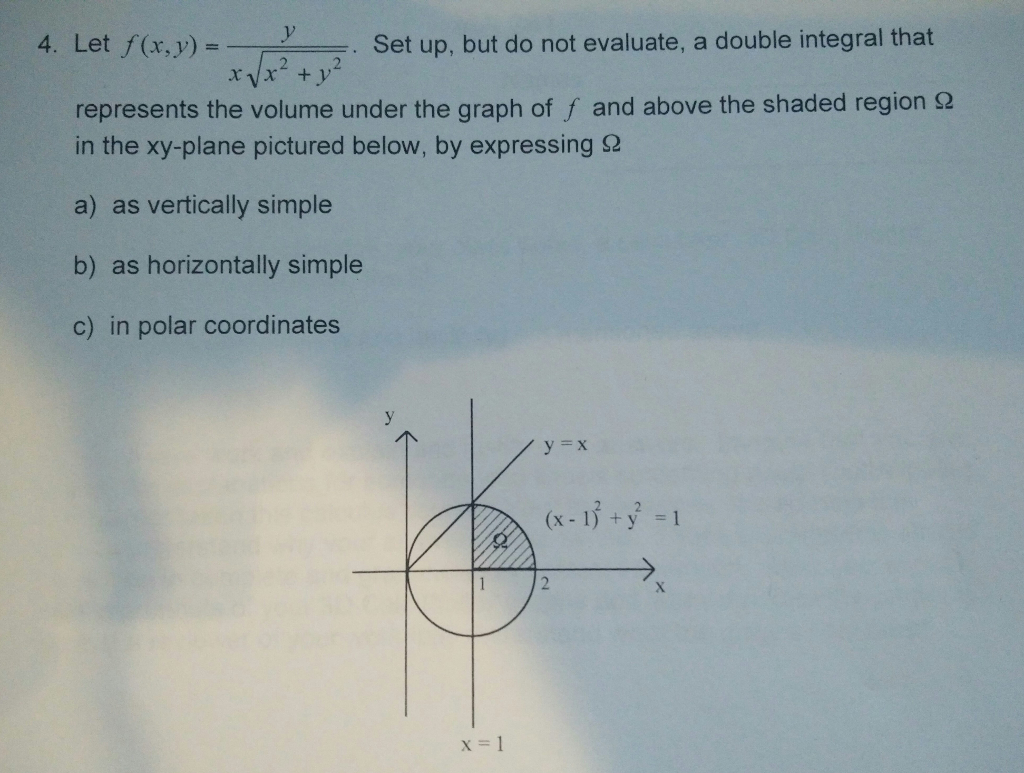


Solved Let F X Y Y X Sqrt X2 Y2 Set Up But Do No Chegg Com



Plzzz Help Asapppppp What Is The Inverse Of F If F X 3 Sqrt X 5 Brainly Com


